Consider the tone row for Chen Yi's "Near Distance" (C, C♭D♭, B♭, E♭, E, G♭, A♭, A, B, F, G, D). The matrix for this row form is:
Notice that the NW–SE diagonal is all the same pitch/integer (here, 0). This is true for all properly constructed twelve-tone matrices. But notice that there is no such consistency in the NE–SW diagonal.
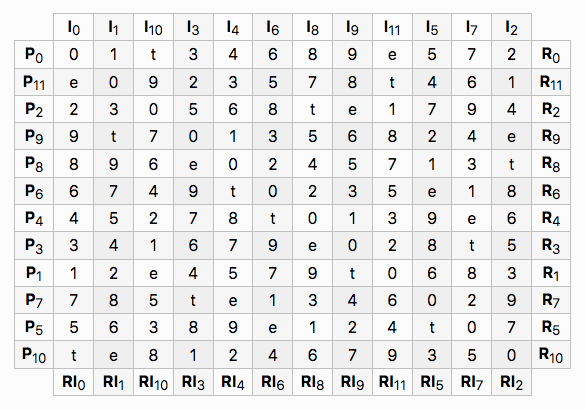
Now consider the row from the "Wittgenstein" Motet by Elisabeth Lutyens (C, B, E♭, G, A♭, E, D, G♭, F, D♭, A, B♭).
This row form is almost symmetrical; the intervals moving forwards are the exact same as the intervals moving backwards, with only one exception: the interval between the sixth and seventh members of the row is a descending major second moving forward but a descendingan ascending major second moving backwards. Because of this symmetry, which ends exactly halfway through the row form, notice that the matrix has a NE–SW diagonal that is "t" for the first half and "2" for the second half:
Thus my question: under what stipulations will a twelve-tone matrix have both of these diagonals using only their own pitch? (By "twelve tone" I mean a row that uses all twelve pitch classes once and only once.) Does this occur only when the row form is completely symmetrical, or are there outside possibilities that would also create such a matrix?
By the way, I'm using musictheory.net's matrix calculator for these matrices.