Db in realization of "A7alt" in Levine's piano book

Question

In this other question about Mark Levine's jazz piano book, there's an enharmonic spelling of b9 of G7b9 as G# instead of Ab, which some users objected to:

To me that seemed completely fine, because it illustrates a common trick I've heard from jazz players of taking a triad (or other chord) found on a half-whole diminished scale and moving it up or down by three semitones:

The combination of these moved chords outlines the complete diminished scale, and it is used over dominant chords. For G7, you would use the G half-whole diminished scale. It's a great trick and it's straight-forward to apply it on different instruments.

Since Levine seems to be aiming his books as practical advice for players, instead of theory people, I thought that spelling of an E major chord is actually correct for playing. And I'm thinking, if there's a surprising enharmonic spelling, it must be because of a practice used by jazz players I'm just not aware of. That "move a chord in steps of minor thirds" trick I happened to know. Layering things thinking about other keys, utilizing chromatic "planing" and other similar tricks are used specifically in order to make the ear's pattern finding start to get ambiguous feelings about the harmony.

However, that's not all. In the beginning of the book, chapter 1 (available here as a free preview chapter) there's a realization of "A7alt." that has a Db:

This D flat is so glaring and bizarre, I can't believe it was accidental. Anyone knows that the third of an A major is C#. And this is right on page 2 of the book. My question is: what sort of practical explanation is there for thinking about a Db when the lead sheet has an A7alt chord? Some practical shortcut or rule-of-thumb pespective that someone might employ during playing?

Answer 1

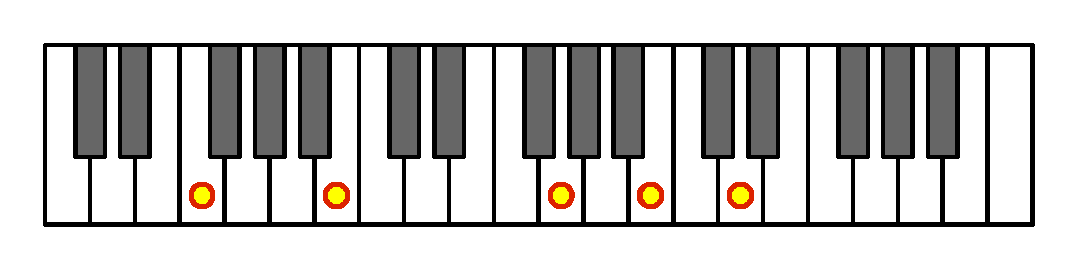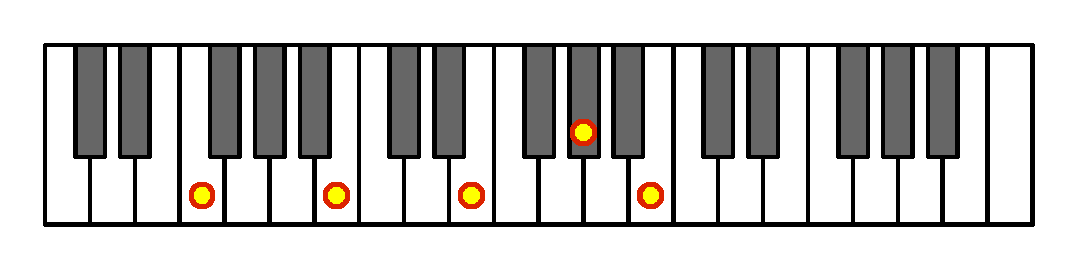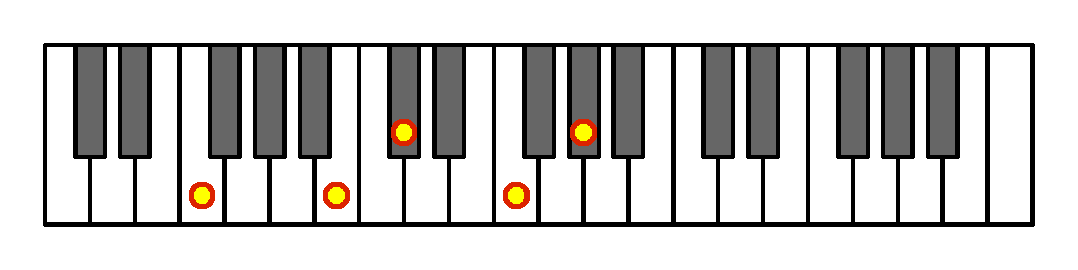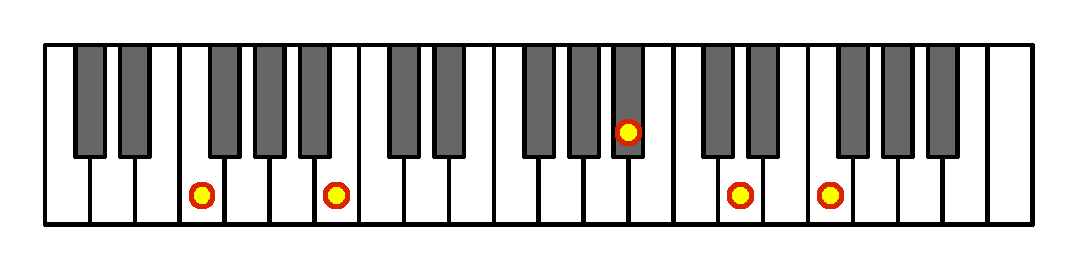
Thanks to @OwenM for pointing towards the solution in the comments (that were subsequently moved to chat). With that I was able to find an explanation in the book itself, in chapter 9, Figure 9-24, which "compares the B Mixolydian mode of E major with the seventh mode of C melodic minor".

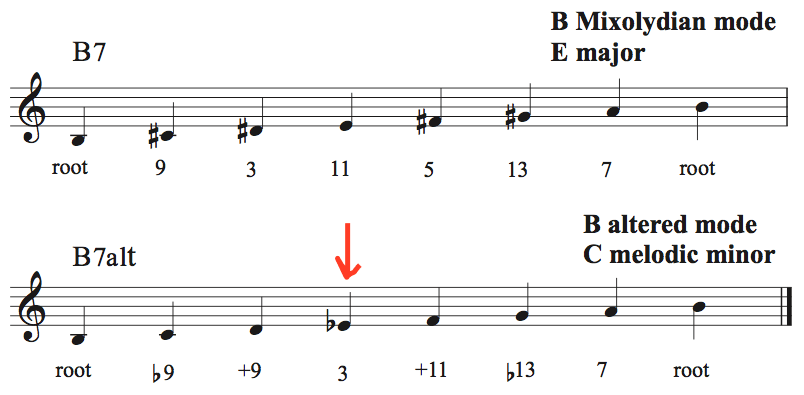
This represents "chord=scale" thinking, where the player associates a chord with an entire seven-note scale, from which the chord is thought to "come from". In the example above, Levine equates the B7 chord with the B Mixolydian mode and takes the note spellings from the E major scale. And he equates the B7alt chord with the B altered scale, using spellings from C melodic minor.

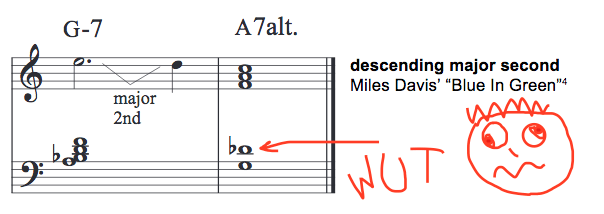
Levine writes that "the eleventh can’t be lowered, it would then become the major third"... Yet in a sense he has indeed lowered the eleventh (E), which has become the major third of the chord.

Problem solution

So, in the A7alt chord, he has taken the note spellings from the Bb melodic minor scale.

He wants to see a Bb melodic minor ("jazz minor") scale on the keyboard with just the root moved to A, rather than an A something scale with lots of individual alterations. This avoids the problem of having (to write? or to think about?) both a flat 9th and a sharp 9th relative to A. Look at it as Bb melodic minor, and then every scale degree gets its own staff position. Whichever way you write it, it's always an approximation and favors one perspective over others.

... but other examples may follow a different logic

If you look at page 3, there's an "E7alt" chord where the same logic has NOT been applied. In the realization of that chord, Levine has written a G# instead of the Ab that he should have gotten from the F natural minor scale. This can mean either that (a) it was a mistake and he forgot to follow the logic, (b) or he imagined a different scale in that case. On page 80 there's some discussion about enharmonic spellings and different perspectives to 7alt chords, and as an additional view he mentions a tritone substitution, which again can change the spellings, if you think that way. The book explains that there are many ways to look at the chords and scales, and none of them is clearly the only correct way.

Is this a music theory book?

If music theory is considered as descriptions of the musical practices of some people, described by someone, for someone, then Levine's book is a theory book. Levine describes the practices of a certain group of people, using a certain style of describing. Both the practices and the description style may not be what you're used to. You have to take it like any human communication - subjective and context-dependent. Adjust accordingly. It must be said that Levine doesn't make understanding him too easy. This book should definitely come with a better how-to-read guide.

How to read the examples

From what I can see, there are two worlds, or coordinate systems in Levine's book. Or spaces? Traditional harmony space and keyboard space? The chord symbols come from the traditional harmony world, where the third of B7 is D#, etc. But on the piano keyboard, Levine imagines a scale that's based on C melodic minor, even so much that he takes the note spellings (even though no actual key signature is written) from there. Just the root note has been moved to the chord's root. The sounding note that in the traditional world is called D#, is played with the keyboard world's Eb note. In this chord=scale, but in some other chord=scale, it might be D# again, I guess. Or whatever scale Levine was seeing on the keyboard when writing the notation.

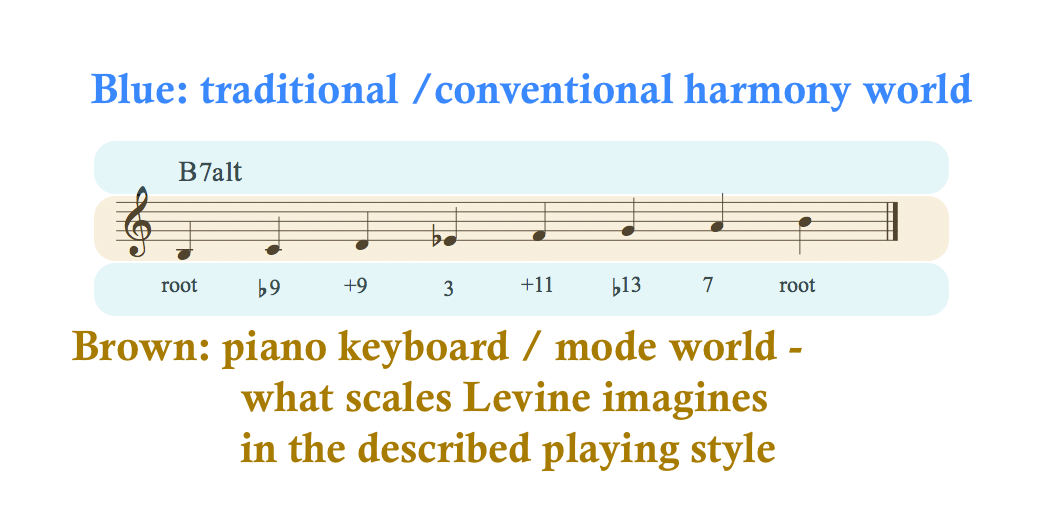
In this way of playing, every chord symbol represents an entire scale for the player. In more traditional harmony thinking, a chord is just its sounding notes, and for the rest, things are left open. But in Levine's "chord=scale=mode" world, every chord symbol means a mode. Which mode you imagine for a chord symbol, is subjective and up to tasted. And maybe that's why the enharmonic spellings can be different case by case - Levine was just imagining something slightly different that time.

Are there people who have read the book more thoroughly? Did I get this right?

Answer 2

...what sort of practical explanation is there for thinking about a Db when the lead sheet has an A7alt chord? Some practical shortcut or rule-of-thumb pespective that someone might employ during playing?

There isn't one.

I think you're proving the point I made in comments on Why is this a G7b9?

Just keep reading the book, you will find more examples of these enharmonic mis-spellings.

Keep in mind the irony that these mis-spelling are presented in chapter one of the book titled Intervals and Triad. The intervals chapter mis-spells intervals! Some enharmonic equivalent intervals are presented with correct spellings, but chords given with jazz labels are mis-spelled and the triads section is presented after a long list of examples using rootless voicings and altered dominants.

The cart is before the horse. It's a marvel of disorganization.

I disagree the book is intended as "as practical advice for players" and not theory.

From the introduction:

Although the Jazz Piano Book is meant primarily for pianists, other instrumentalists can use it as well, both an an introduction to jazz piano and as an aid to understanding harmony on their own instruments...much of this book involves music theory...

It's clearly meant to be practical and theoretical.

Also, regarding the idea these mis-spellings are deliberate for the sake of reading and practical performance: these are not jazz charts. These are supposed to be basic examples in a textbook. Additionally, there are numerous examples of transcribe performances excepts. Those too are not presented as charts for performance.

Yes, you could play the music examples from the book, but that doesn't mean they are performance material. It's meant primarily to be read. How else would you present the ideas in written form other than staff notation? If it were a guitar book, it could be all in tab, and the enharmonic mis-spelling issue wouldn't come up. But there is no common tab system for piano. Staff notation is piano's written medium. For the sake of musical readability the book ought to use proper chord spellings.

I only use the book because I see it widely referenced.