Question about dissonance handling and counterpoint

Question

I'm self-learning 18th-century music theory because I like the music from that era. I'm obscure about the concepts of counterpoint and harmony. I'm aware that rules are not absolute within the 18th-century music. I heard the music adorned with augmented seconds. So I have a few questions.

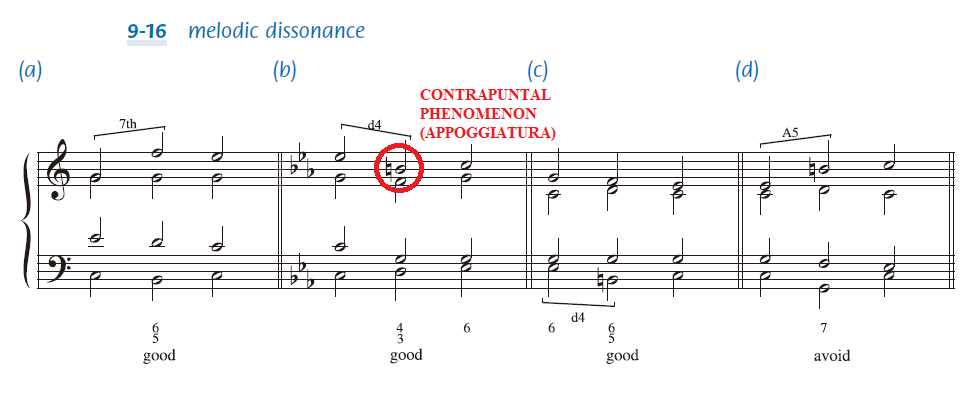
In 18-century harmony, there are two distinctions: structural chords and prolonging chords. In this case, it appears that such dissonant leap usage is permitted as a prolonging chord.

1. In the case mentioned earlier, the prolonging chord dominant inversion is singular, but, it seems possible to prolong the chord even further than this. So, I'm unsure about the condition for allowing melodic dissonance in the strict composition. Is melodic dissonance only permissible when it's singular, and is the concept of prolonging chords broader?

I'm also struggling to grasp the concept of 'seventh.' It seems there are situations where the concept of 'seventh' is necessary. When looking at this phrase, explaining F# as a suspension resolved to E doesn't seem to fit:

In such cases, there are several cases where it's not inappropriate to refer to a note as a suspension without invoking the notion of 'seventh':

2. I've also learned that seventh should resolve after preparation like any NCTs, so I'm not sure how to distinguish between them.

I have these questions, leading me to the following inquiry.

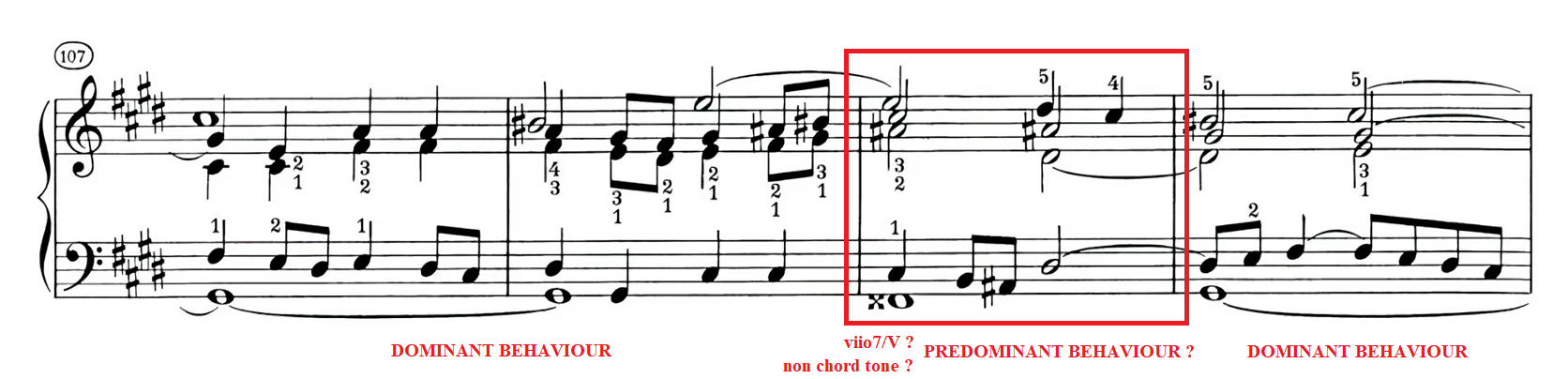
So, it may sound peculiar, but when listening to fugues, it seems that both non-chord tones and chord tones can coexist within a single chord. In other words, it appears that there are instances where the melodic dissonance and chordal dissonance do not align. Here are a few observations I can make in this case:

• The chord of first beat of measure 109 is a structural chord.
• If the answer of 1. is restricted to only 'singular,' then either B# or E of measure 108 must be a prolonging chord. In other words, either B# or E is contrapuntal phenomenon. (in a broader perspective, it is currently within the dominant domain)
• To explain the chordal dissonance between Fx and E, either G or E on beat 2 of measure 108 is a chord tone.

then, possible explanations could be E is a suspension and Fx is not contrapuntal, or Fx is a neighbor tone and E is not contrapuntal. However, if E is indeed not contrapuntal, it raises questions regarding the conditions for B# to be contrapuntal in the context of melodic dissonance, or whether melodic dissonance and chordal dissonance should be considered separately.

I have these questions, and therefore, I'm unsure how to perceive dissonance.

Answer 1

The first example: There is nothing special about the example you show, certainly not in the soprano. The leap by a tritonus is very common and just standard 3rd-7th-1st progression in minor tonality by the rule of the octave in the soprano. In the bass, it's even more standard 1st-2nd-3rd.

The only unusual thing is the fact that the F is not resolved to Eb, which would be the proper thing -- while it is by a full tone, the role of the F as a leading note should not be ignored. This can be explained by fauxbourdon (parallel 6th chords), but it is slightly non-standard, in particular if the Eb isn't in the same position in another voice.

Now a bit more general: You can't really separate the "harmonic" and "ornamental" function. It helps when you start, but I have a feeling from your first call of the B natural as "ornamental" that you already confuse things a bit. So I'll offer another way of thinking: Each note has "a" function, and the note can be where it is if you have a way of explaining it by "a" function, regarless of what function it is. The confusing thing could be that both "appoggiatura" and "leading note" have a similar function -- they create a tension, and we have a good feeling of how that tension shall resolve. (Note that this means that if you don't resolve it the expected way, it's fine, as long as that lack of resolution plays a function, maybe to surprise the listener -- see how it complicates?)

Second example: That's a nice and a bit complex one. If it were a four-fold cadence into C#, I would say it's simply G# with figures 7 5 (3) -- 6 4 -- 5 4 -- 5 3, which is a common variant. But here it seems modified as (8) 7 5 -- (8) 6 4 -- (9) 6 4 -- (3)=(10) 7 5 and who knows where it goes. (I actually love this idea of 8 6 4 -- 9 6 4 and I shall implement it at some time as it's so cool!)

Thinking about it in terms of "7th chords" (in the modern way of chord naming) is so limiting that I would recommend avoiding that and just thinking about it as a very nice cadential progression over the penultimate!

Third example: Offers two explanations. You seem to explain the Bb as a 7th over the C that resolved to a 3rd of the F. This is probably more plausible given there is already a C major chord before that Bb, but you could also explain it as a suspension of the C in the 16th, so as ornamentation. (See how it gets complicated?) The rest is funnily simple: F 7, F# 7b as a sharpening, leaping to Bb major -- that's quite courageous, but why not :)

Fourth example: First, I would avoid using marks such as viio7/V, that's so limiting compared to the contrapunctual richness of the music! But if you insisted, then I would say it's F##7 (yeah, diminished etc., but that's not that important) -- G#7 -- c# progression, basically just subdominant -- dominant -- tonic, with the subdominant being enriched with a 7th instead of the usual 6th, the rest is just ornamentation. Bar 110 is then again a 7 5 -- 6 4 cadence. If you asked what role does bar 108 play, then it's just a dominant that's not resolved to a tonic but rather prolonged by sidestepping to the subdominant, basically making one very long penultimate step. (The function of this sidestep is to enforce the dominant nature of the G# bass note.)

If you wanted to be precise, then I'd say that structurally it is[108] G# 9 7 - 6 4 | [109] F## 7b 5(b) -- 6 -- 6 5(b) | G# 7 3#, but maybe some of the numbers in bar 109 can be taken as ornamentations. (I would be reluctant to call the E as an ornament though, as it's doubled by the end of bar 108, suggesting that one of them is a prepared note, really belonging to the first chord of bar 109, where it then probably should be considered as structural. But who knows.)

Conclusion: I'm not sure I helped, but I hope I at least managed to show the complexity and maybe a (non-unique) way of thinking about the music. TBH, if you are new to the topic, you didn't choose some simple music to start with.

Answer 2

For Roman numeral analysis, the second half of that measure is V7/V. The F double sharp is definitely not a non-chord tone. Bach would have thought of it as a 7-6 suspension between the soprano and bass.

From the comments:

If that's a suspension, then from my perspective, it seems reasonable to view it as "decorated resolve" at measure 105, beat 2.

I'm not familiar with the term "decorated resolve," but it is certainly a resolution, and it certainly is decorated, so I suppose so.

I started to separate the terms "non-chord tone" and "contrapuntal." If we think completely contrapuntally, whenever there's dissonance between two voices, one of them should be contrapuntal.

If we think completely contrapuntally, how can anything be not contrapuntal? What would it mean for a note or a voice to be not contrapuntal?

Thomas Benjamin 'The Craft of Tonal Counterpoint' suggests that Fx of measure 109, beat 1 is a neighbouring tone.

Well yes. But it's not a non-chord neighboring tone (as is the D♯ in the "decorated resolution" discussed above) because the harmony changes.