9

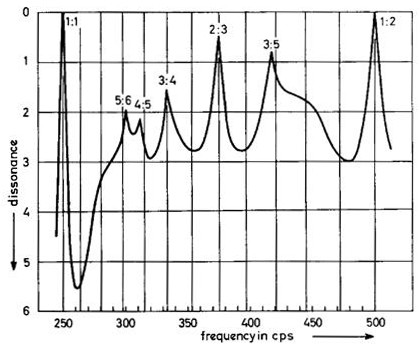
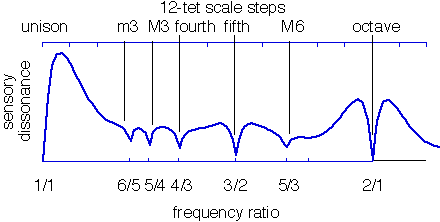
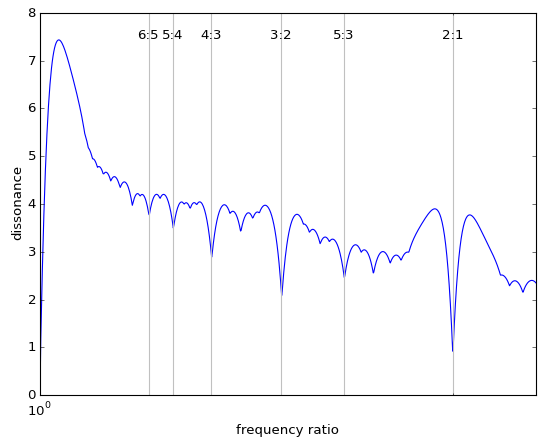
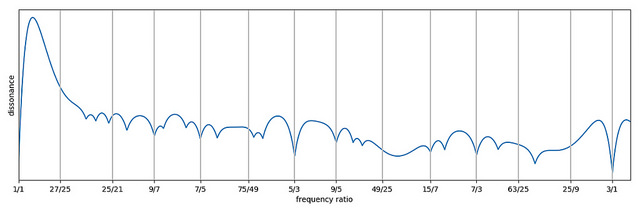
Roughness is explained well in Is there a way to measure the consonance or dissonance of a chord?

In particular the Plomp-Levelt curve is derived, which has various dips showing how simple intervals (3/2, 4/3 etc) are less rough than the average.

However the curve appears to have no dips for the interval 7/6 and above.

Why is this?

Is it because the curve is empirically derived, and human perception cannot (on average) distinguish these intervals from arbitrary ones?

Or is it a limitation of the model represented by that curve?

Improve this question
3
  • I suspect part of the reason why the Plomp-Levelt curve has no dips there is because we generally consider minor thirds to be more consonant than major seconds, which are themselves more consonant than minor seconds, and so on. What's also interesting about that curve is that apparently the tritone(/augmented fourth/diminished fifth) is still considered as somewhat consonant (can someone confirm whether it's more consonant than the minor second according to that curve?).
    – Dekkadeci
    Commented Dec 27, 2017 at 8:26
  • 1
    Good point. According to that curve any interval between major second and octave is considered less rough than the major second, and almost any interval is considered more consonant than minor 3rd. That really doesn't seem right to me - is this the impact of cultural training?
    – Sideshow Bob
    Commented Dec 27, 2017 at 11:50
  • @Dekkadeci the Plomp-Levelt model calculates less beat interference for a tritone than a minor second. I would hesitate to apply consonance directly to that result, as consonance could be made up of other factors besides beat interference. David Cope in "Computer Models of Musical Creativity" (p.229-230) also ranks the tritone as having lower tension (0.65) than the minor second (1.0, maximum) or major second (0.8).
    – thrig
    Commented Dec 27, 2017 at 19:30

1 Answer 1

Reset to default
15

If you mean this curve:

Plot of dissonance vs frequency difference, showing peaks at 1:1, 5:6, 4:5, 3:4, 2:3, 3:5, 1:2 frequency ratios

probably because it was only calculated using the first 6 harmonics.

Plomp & Levelt 1965:

In this way, the curves ... were computed for complex tones consisting of 6 harmonics. ... shows how the consonance of some intervals, given by simple frequency ratios, depends on frequency.

And this one:

Sethares consonance curve with 6 harmonics

was also only calculated with 6 harmonics.

7 harmonics would produce a notch at 7:6.

I also did a curve with 14 harmonics, and it has a notch at 7:6 and lower:

Sethares consonance curve with 14 harmonics

(and here's one with all audible harmonics)

Timbre and odd vs even changes the curves a lot. Including only odd harmonics produces notches at some of the intervals on the Bohlen-Pierce scale, etc:

Sethares dissonance curve for tones with only odd harmonics, 1 through 17, along with vertical lines at intervals of Bohlen-Pierce scale.

Improve this answer
7
  • Thank you for those graphs. The first two show no notch for 8:5 (minor 6th) even though that shouldn't be markedly less consonant than minor 6th or minor 3rd. Is it really objectively less consonant than conventional musicology claims, or does this show a fault in the model?
    – Rosie F
    Commented Mar 21, 2018 at 7:14
  • 1
    @RosieF It's uncommon for an instrument to have 6 harmonics and then abruptly stop, so I'd say it's just an artifact of the way these particular graphs were made. One could generate such tones and listen to a sweep in the vicinity of 8:5 and see if one hears an especially consonant point or not. (And by "one", I mean "Me, but I don't have time right now")
    – endolith
    Commented Mar 21, 2018 at 15:53
  • @RosieF At that interval the frequencies would be 1 2 3 4 5 6 and 8/5, 16/5, 24/5, 32/5, 8, 48/5, so there would be no harmonics in common.
    – endolith
    Commented Mar 21, 2018 at 21:24
  • @RosieF Nevermind I made the sound file: soundcloud.com/endolith/6-harmonics-sethares-plot
    – endolith
    Commented Mar 21, 2018 at 21:51
  • Hi Endolith - any chance you can share the details of how you produced your 14 harmonic curve?
    – Нет войне
    Commented Jul 16, 2019 at 23:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.