In minor, can the neapolitan sixth chord resolve to the diminished seventh chord on the leading-tone (of the same key)?

Question

What would that look like voice-leading-wise? I'm assuming more or less traditional common-practice harmony rules. Examples from the literature would be much appreciated.

Answer 1

My hunch is that, if a ♭II6 did resolve to vii°7, it would tend to resolve to one in second inversion (that is, vii°43). This way, the bass scale-degree 4 stays constant. This is one reason why ♭II6 resolving to V42 is so common.

I know of two examples in the literature where ♭II resolves to vii°7. First, and I think most in line with what you're looking for, is from the opening measures of Saint-Saëns' "Organ" Symphony. Here, the ♭II6 resolves to vii°65:

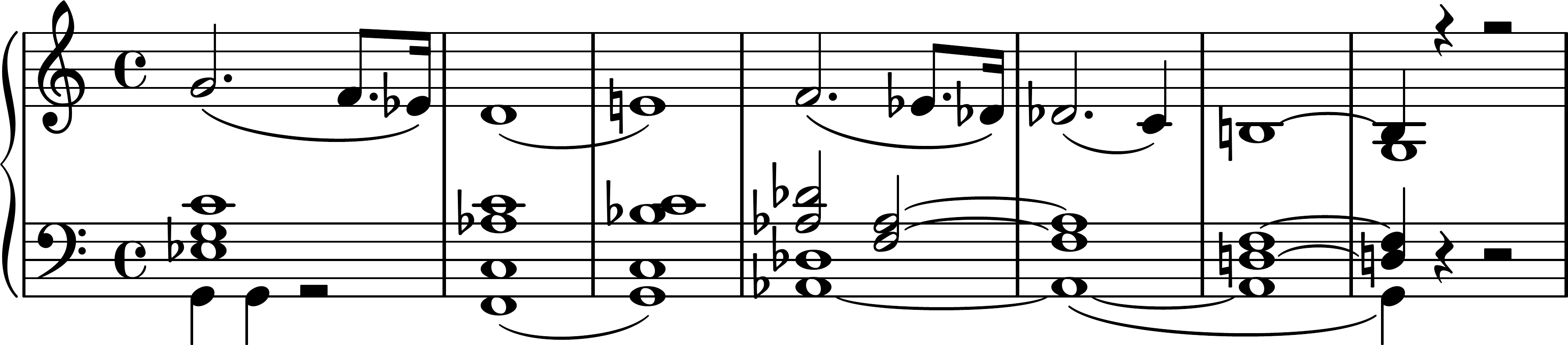
Another example to consider is from Weber's Overture to Der Freischütz.

There are a few things to notice here:

1. The Neapolitan (mm. 4–5) is in second inversion (!).
2. It resolves to a vii°7 chord in third inversion (m. 6).
3. But most importantly, that vii°42 really just delays the appearance of V7 on the downbeat of the last measure. In other words, I view the A♭ in the next-to-last measure as a suspension from the ♭II64. As such, I hesitate to call that measure a vii°42; in my opinion, the final two measures are V7, we just have a long suspension in the bass.

More common, as you probably already know, is for the Neapolitan to resolve to an applied diminished-seventh chord of the dominant. As one example of many, consider this quick example from the last movement of Mahler 2:

The Neapolitan is on the third beat of the first measure, and it moves through vii°7/V on beat 4.