Why are only even values used for note duration?

Question

So, I was wondering why there are only even numbered notes? Like half, quarter, and eighth and so on.

Answer 1

So, I was wondering why there are only even numbered notes? Like half, quarter, and eighth and so on.

Because, O my child, we are a weak, degenerate people. Because we live in an Iron Age, not like those who came before. Not like before, in days long past, when musicians strode the earth like giants of artistry.

They divided notes into thirds.

This has been how you have been taught: that the basic unit of musical time is a quarter note, and that you build up rhythms by combining quarters, halves, eighths, sixteenths and the occasional whole, like they were different sizes of musical Lego(tm) bricks. You were taught to think of rhythm as additive.

But about a thousand years ago, when musician-scientists were first figuring out how to write down rhythm such that the reader had any hope of decoding what was meant, they thought of rhythm in a very different way.

If you were to ask, oh, Perotin in 1198 AD to explain rhythm, he might say to you, "Look here, music is just like poetry, so we use the poetry terminology from Classical antiquity. For convenience, and because we're big old geeks, we've numbered them. The first rhythmic mode is the trochee: long-short. So music in the first rhythmic mode goes DAH-dah DAH-dah DAH-dah etc." And he'd be singing what we'd notate as quarter, eighth, quarter, eighth, quarter, eighth. Which is to say, what we expect to see in 6/8.

Perotin would go on to explain that mode #2 was the iamb: short-long. Also ternary. But wait! (you might ask, remembering your metrical feet from high school poetry class) What about the dactyl? Isn't that long-short-short? "Yes!" beams Perotin "That's number 3. DAH-da-dah!" Dotted quarter, eighth, quarter, or what we call 3/4.

Binary time in the rhythmic modes is down at #5, the spondee. But it's notated as 6/8 because while it's pairs of longs, they are further subdivided as triplets.

In the late 12th century, ALL the "time signatures" they had were ternary on some level.

(As an aside, because pretty much everybody with access to a pen and ink was clergy, there's apparently contemporary argument that this whole "divided by three" thing had theological significance -- you know, the Holy Trinity -- but that has more than a little of the whiff of post hoc rationalization.)

Listening break! Here's Perotin's Viderunt Omnes from 1198 over the original notation. (Prefer modern notation? Here.) Trivia: first four part piece in the historical record. TTTB.

Our SE founder, Joel Spolsky, once quipped that the great thing about Wikipedia is that if he ever wanted to know something that he couldn't find in Wikipedia, he could just start a new page about the topic and put something down completely and obviously bogus, and somebody would come along, be incensed, and correct it, thereby answering his question. Whenever I think about rhythmic mode notation, I am reminded of that, because while you have to give those guys credit -- the rhythmic modes were the first real attempt to get rhythmic notation off the ground -- it was the absolutely terrible solution that drove the invention of something that actually, you know, worked.

And that was mensural notation. Our irritated Wikipedian-analog was Franco of Cologne, who gave us the crucial innovation that mensural notation was based on: that duration should be encoded in note shapes -- what we do, still to this day.

However, they still thought of musical rhythm from the top down. So in medieval mensural notation, and the variants descended from it for quite some time, rhythm was defined in four layers. The tempus (Latin ="time") of a piece was how many semibreves a breve was divided into. A complete breve was one that had all three counts. Because, clearly, the right, full, correct number of subdivisions for a note to have is three. The Latin for "complete" is perficio, or as we would say (and is still an archaic but used definition of the word in English) perfect. A breve that only gets two semibreves, clearly, is incomplete, and thus imperfect. Thus we can say of a piece where breves are to be subdivided by three that it is in perfect time, while one in which the breves are only divided by two is in imperfect time.

(I'm not making this up. In early music, we actually describe music as in perfect and imperfect time.)

But that's just one level. The semibreve, itself, might be subdivided into either three (perfect) or two (imperfect) minimums. This level was called prolatio, or as we say today prolation. With both tempus and prolation, we have the makings of a basic rhythmic vocabulary. We can say of a piece that it has perfect time and perfect prolation -- three semibreves to each breve, and three minims to the semibreve, or what we'd notate as 9/8 -- or perfect time and imperfect prolation -- what we'd notate as 3/4 -- or imperfect time and perfect prolation -- what we'd notate as 6/8 -- or imperfect time with imperfect prolation -- what we'd notate 4/4.

Additionally, there were two higher layers: how many breves to the longae (this is the modus or mode, and how many longae to the maxima (this is the maximodus). Nobody seems to care about these.

Listening break! Have a Franconian motet, so called because it was notated in the then-new notation of Franco of Cologne. Okay, back to work.

Over the next, oh, three hundred years, music in imperfect time with imperfect prolation slowly became more popular, moving from something about as unusual as 9/8 is today (i.e. totally legit, its just nobody ever much uses it), to... well, I just eyeballed my copy of Odhecaton (pub 1501 AD) and it, of its approximately 100 pieces, has fewer than 10 in ternary times. Peri's Le Varie Musiche (pub 1602 AD) is about 75% binary time.

By the time you get into the 17th century, the roles of binary and ternary time have pretty thoroughly switched. Still, the basic notation system persists, and people are still using the terms "breve", "semibreve", "minim" and so forth.

Here's the part where I get hazy, because, really, if it happens after 1651 and before 1960 I simply don't care. But what I have been able to discover is that the American English terms "half note" and "quarter note" and all the rest, which presuppose binary divisions, came from German into English in the 19th century. So somewhere around there, or previous, ternary time music has become rare enough that it had become natural to consider the subdivisions of the breve and the semibreve into two parts the default and natural order.

And that is how it came to be that Americans (an those who learn our musical terminology) came to refer to one sixth of a bar counted in six as an "eighth".

P.S. One last listen: the 16th century was, as far as I'm concerned, the last great gasp for ternary music. The 16th century saw a hundred year vogue for the hemiola syncopation, which became to dance music of the end of the century what swing was to the mid-twentieth's dance music. The hemiola is when you play 6/8 and 3/4 at the same time. Of course, you can stack them too. The Fairie Round by Holborne, 1599. (Score)

P.P.S. Update: It occurred to me to pull out my copy of Yudkin's translation from the Latin of the "Music" chapter of Freig's Paedagogus from 1582 AD. It says this (yes, it's in the FAQ format so beloved of late 16th cen authors):

How many kinds of note-lengths are there?

Two kinds. The notes are either longer or shorter than a semibreve (which is what determines the mensuration).

Which are longer than a semibreve?

The brevis, the longa, and the maxima. A brevis is two semibreves, a longa is four, and a maxima eight.

Which are shorter than a semibreve?

The minim, semiminim, fusa, and semifusa. Two minims, four semiminims, eight fuase or sixteen semifusae are equivalent to one semibreve.

How many knids of count are there?

Two kinds. Duple and triple.

What is duple?

When one semibreve, or two minims come into one count. That is called tempus imperfectum and the sign is [our "cut time" sign].

What is triple?

When three semibreves or their equivalent are sung in the time of one semibreve. And this is called triple proportion, or tempus perfectum, and the sign is [sign: circle with dot in it] or [sign: circle]. This proportion is twice as fast as taht which is normally called triple....

This is fascinating because he clearly thinks of note values as we do: two eighths to the quarter, two quarters to the half, and so on. That is his default, basic answer to the question of what note values there are. But at the same time, he still thinks of measures in the old way, of duple and triple time, and goes right on to describe triple time as that in which there are three half notes (semibreve) to the whole (breve).

Answer 2

So, I was wondering why there are only even numbered notes?

Because they add up. :) Two eighth's make a fourth; two fourths make a half; two halves make a whole, etc.

However, there also notes that do not follow this binary pattern. They are called tuplets. The most common form of a tuplet is a triplet. This is a note divided into three equal sections. There are also duplets, quadruplets, pentuplets, etc.

Also, because it's all about note duration, how do we express the duration of a note, say a whole, on a guitar or any other instrument? Like how many seconds?

To start with, every piece has a time signature. Here you can see the 4/4 time signature. This means that every measure will have four beats, each lasting a quarter note. The top number describes the number of beats per measure and the bottom number defines the type of beat (half, quarter, eighth, etc.).

In this piece, I have written a quarter note on every beat in the upper staff (line of music).

The specific duration of a note is defined by the tempo. A tempo marking is written in beats per minute (BPM). So, 120 BPM means 2 beats per second. This is the same on every instrument.

Often, the tempo is not a specific BPM, but a word, such as Allegro. Allegro means the tempo is roughly 120 BPM. So here, each beat (quarter note) lasts .5 seconds.

However, if the piece were marked Adagio instead of Allegro, the tempo would be a lot slower, about 45 BPM. Each beat (quarter note) would last 1.33 seconds.

Answer 3

"So, I was wondering why there are only even numbered notes? Like half, quarter, and eighth and so on."

I can't say why it evolved this way. However, it does the job. If your question is, how do you create notes that have a duration which is in between these pre-defined values, then there are several extra notational devices:

• a dot behind the note extends its duration by half. So a quarter note (duration is 2 8ths) with a dot has a duration of 3 8ths.
• two dots ("double dot") behind the note extends its duration by half plus half of its half. So a quarter note with two dots has a duration of 7/16ths (1 quarter + 1 eigth + 1 sixteenth)
• notes can be tied to indicate the sound is to last for the duration of the combined length of the tied notes. The tie appears as a curve extending to notes that are immediately adjacent and on the same pitch. Ties can be used to allow a tone to sound beyond the barline, but it may also be used within a measure.
• to work with odd subdivision of the beats, you can use "tuplets". For instance, the indicate that the duration of a quarter note is to be divided into three notes of equal value, you'd use eighth-triplets. This is denoted by writing the number of notes that divides the beat right above the beam that joins the notes. If the notes aren't joined by beams (for example, in case of say triplet quarters), brackets are used to denote the tuplets that form a group, and the number is written halfway the brackets

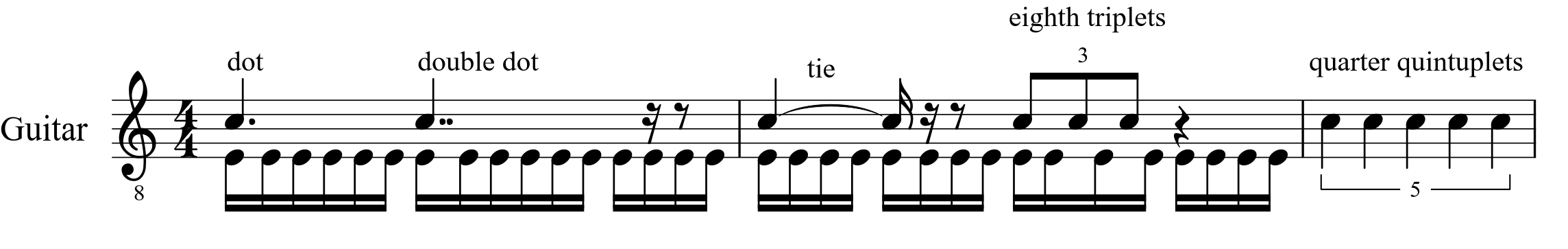
Apart from these devices, which act on the duration (the amount of space in time occupied by the sound of the note), there are a number of articulation markers. These do not alter the duration of the note, but they can specify for how long to sound the note within its denoted duration. For example, a dot right beneath the note indicates staccato, which indicates the sound of the note is to be cut off instead of letting it ring for the full duration of the note. For example, a quarter note with a dot beneath it may sound as an eighth note followed by an eighth rest.

EDIT: parallel rhythms are notoriously hard to typeset nicely in notation programs as notes of different length are not usually typeset strictly proportionally in order to save space. I'm adding a variant from LilyPond 2.19 for comparison: