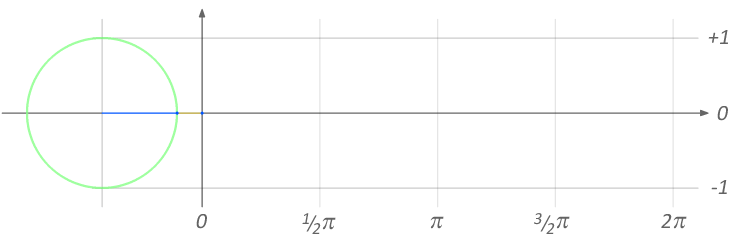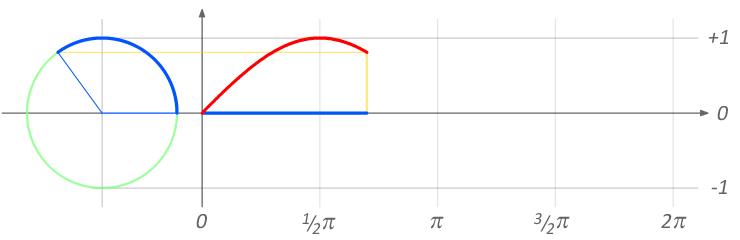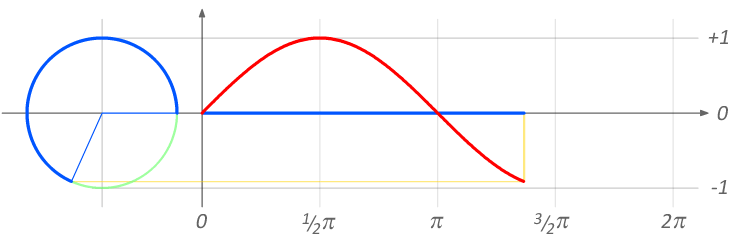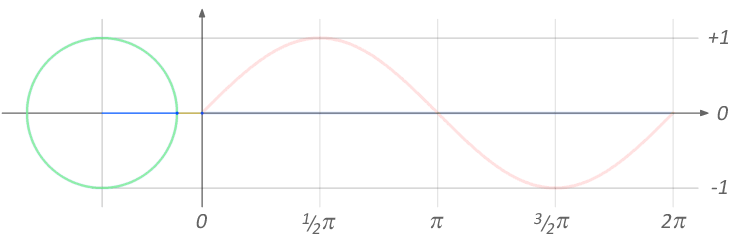Why are pure tones depicted as sine waves?

Question

This is pretty much the only thing in music theory (that i've covered so far) that I cannot understand. What I would expect to hear is what you get from LFO, or Low Frequency Oscillator represented by a Sine wave where the pitch is varied. Here the change in pitch is very smooth because, well, it's a sine wave :D

But then a pure tone is also represented by a sine wave. I presume that when such a wave is plotted on a graph, the x axis is time, but the y axis can't be pitch. You see, it seems that when playing a sine wave, nothing varies but time. However, a cyclic change always represents a change between two variables, indeed a relationship (horay for maths!) So what's the relationship?

Furthermore, I would of thought that the graphical relationship would be the one obtained from a frequency analyser, where you just have one vertical line representing the fundamental (the keyword being one, all frequencies are represented by a vertical line on a freq analyser, x = pitch, y = hertz).

Now in the hopes of not going to off the beaten track, independent of the reason for using sine waves, are there any usages of cosine? Is this just the same thing? Even though a cosine is just a sine wave but shifted, I suppose this wouldn't make a difference, right? How about the tangent, or other functions?

Answer 1

I'm just going to answer the question "What about tangent, or other functions", since the rest seems to have been fairly well handled.

All sounds that we hear as having a definite pitch or note can be represented by a periodic function. As I wrote in my comment, any repeated shape represents a periodic function. Most periodic functions, both in the real world and in theory, are pretty complicated, at least mathematically.

If we want to do math on the sounds we are hearing, we will have to get a grip on these periodic functions. What would really help is a way to simplify them. As discussed, Fourier analysis lets us do exactly that. We can take a complicated periodic function with some really annoying math and break it down into simpler periodic functions where the math is much easier.

The simplest periodic functions are sine and cosine, and they are virtually the same thing and they are closely related. Tangent is a very famous periodic function and is quite helpful in basic trigonometry (literally the study of triangles). Tangent has many other uses, but most people first see the tangent function as a way to analyze triangles.

Even though we usually learn about sine, cosine, and tangent at the same time, the tangent function is actually different from sine and cosine in some important ways. It is not continuous, which means you couldn't draw very much of it without picking up your pencil and putting it down again. It's even worse than that, because it has an infinite number of discontinuities (places where you have to pick up the pencil). Note that not only is this a stark contrast with sine and cosine, but also with periodic functions that model sound waves. Sound waves are continuous functions if we plot them out.

So if we want to take a periodic function and break it into simple pieces, we don't want to break it into tangent pieces. Tangent will not help us, it's really more likely to make things worse. When Fourier (the mathematician who invented Fourier analysis) was trying to take apart periodic functions, he was looking for a way to turn them into sines and cosines, not any other periodic function (and there are many).

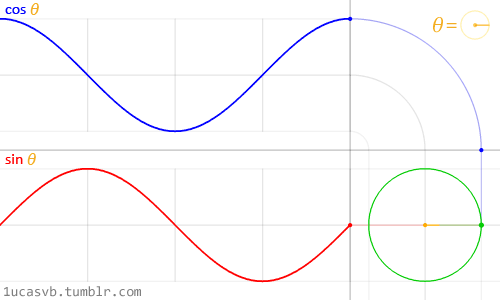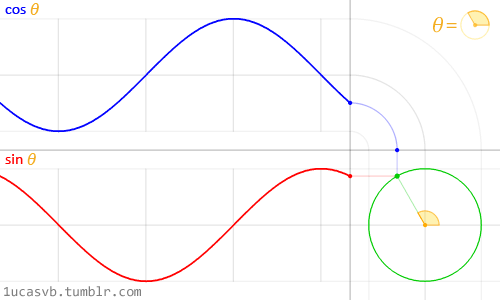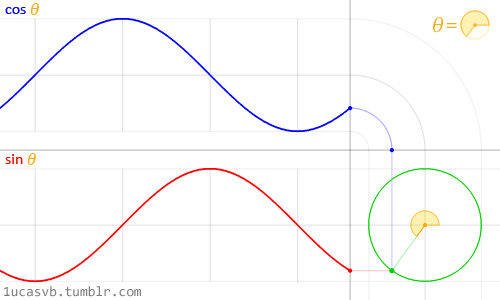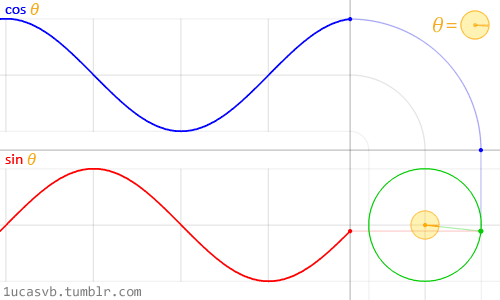
I could write several pages on why sine and cosine are not merely simple periodic functions, there are actually the simplest possible ones, but I don't think this is the best place for that. Let me briefly say, however, that a circle is perhaps the simplest shape, and if you follow a point as it goes around a circle, its vertical movement traces out a sine wave at the same time that its horizontal movement traces out a cosine (this depends on where on the circle the point starts, but one will be sine and the other cosine, or at least one will be one quarter cycle behind the other in phase).

Below is a video of that, and hopefully this will give you a way to see how the sine wave (or cosine, same thing, really) is the simplest periodic function, and therefore the one we want to break more complicated functions into, and also the one that to our ears appears to be the most basic if we turn it into sound.

See also the Physics version of this question with a similar answer: https://physics.stackexchange.com/questions/352754/why-cosine-and-sine-functions-are-used-while-representing-a-signal-or-a-wave

Answer 2

Sine and cosine are the same, just offset by 90 degree. They form a "quadrature pair": if you add their squares, you get a constant. When you draw a sine wave as a representation of audio, it represents either pressure (compared to neutral) at some "listening" point, or an impulse density. Both together form a quadrature pair again: if you square and add their respective sizes for a stationary sine wave noise, you get a constant representing the sound energy density.

Now why sine waves? Some people state that's because you can let everything be composed of sine waves but that's circular reasoning: almost any wave shape can be used as a basis for decomposing signals: one can easily represent a sine wave as a composition of an infinite number of square waves just like it is possible to do the reverse.

However, what singles out sine waves is that they are the Eigenfunctions of linear time-invariant systems (which are the vast majority of systems working on sound: any ambient surrounding without some loose snaring part, amplifiers as long as they are not overdriven, delay circuits, equalizers and a number of others): if you feed such systems with a sine wave, the output will be a sine wave of the same frequency though possibly different volume and phase.

No other wave form has that feature: square waves don't remain square when fed through such systems, sawtooth waves don't remain sawtooth, frequency sweeps of constant amplitude change into sweeps with varying amplitude.

There is no such thing as a "muffled" sine wave from behind closed doors: it may be attenuated but its sound quality is totally the same.

Answer 3

Because a circle is the purest periodic shape:

Any periodic orthogonal function could form the basis of more complicated, "less pure" sounds (i.e., sounds with many harmonics). See Dave Benson's excellent (and free!) Music: A Mathematical Offering (Cambridge U. Press), ch. 2, "Fourier Theory."

Answer 4

I like Darren's beginning very much, converting your question to "Why do we perceive sine waves as pure tones?"

When the wave that is traveling through the air reaches the ear drum, the ear drum vibrates. That vibration is transferred to the three little bones in the middle ear. To make a long story short, the next-to-last step in achieving hearing is that the fluid inside the snail-shaped thing goes forward and back just as the air molecules did while the sound wave was going through the air towards your ear. Now, inside the snail-shaped thingie, there are a large number of little hairs attached to the "floor" or the "wall." They sway with the vibration that was communicated from the middle ear. They oscillate, like a sine wave - forward and back, forward and back.

The frequency of oscillation gives rise to different pitches.

Answer 5

To supplement the impressive mathematical background exposed in the other answers (kudos to Geremia's link), I want to summarize my own, to get two more practical aspects in:

• A tone is decomposed by our ear/brain combination into a base tone and overtones. (That other decompositions are also theoretically possible has therefore no impact). The overtones add what is called timbre. Obviously one achieves a quite pure tone, if the timbre is removed. For direct experience listen to a sine generator, or lacking this, to a flute, which is quite close to sine and in contrast to a clarinet, which is far from it.

• A sine wave depicts a harmonic oscillation. I now have to resort to a mechanical wave. A harmonic oscillation is an undampened oscillation, where the restoring force is proportional to the equilibrium offset. For a pendulum this means proportional to the angle to the vertical. There is no simpler formula than direct proportion, therefore sine wave is the most simple wave.