How do I harmonize a C major scale in fourths and then apply negative/mirror harmony?

Question

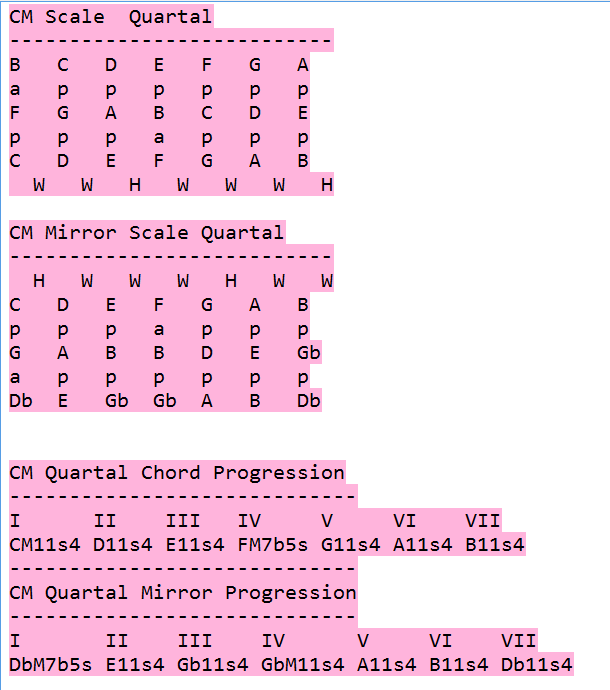
I recently tried using quartal harmony and mirror harmony (also known as "negative harmony") together. I was looking for some music theory that would inspire me to do something more unusual than I usually do.

I took the C major scale, harmonized each scale degree diatonically in 4ths, and then mirrored it. If I may, the end result sounds very jazzy (though I have no idea what jazz is).

Here is the scale, harmonized and then mirrored. I acquired the chord names using software.

Two notes about notation: in the chord names, the "s" stands for suspended and the "M" stands for major, as in D♭Maj7♭5sus. The "p" and "a" between notes in the harmonizations stand for perfect fourth and augmented fourth, respectively.

Is my resultant chord progression correct? Is this the correct way to harmonize a C major scale with quartal voicings and then apply the mirror harmony?

I suspect there is an error because the final progression has two pairs of chords with the same root notes, D♭ and G♭. That seems strange to me. Could anyone point out if I made a mistake? I am by no means a music theory buff, nor do I have musical talent.

Answer 1

You are starting with:
        quartal voicings that ascend up the C major scale

The result you are looking for is:
        quartal voicings that descend down the C phrygian scale

Your flaw is that you've continually changed the "reflection point." Here's the process to follow. Your error is in step 6.

1. Start with the 1st degree of the C major scale.
2. Build a quartal chord off that scale degree: C-F-B (ascending).
3. Construct a mirror chord, using C as the reflection point: C-G-D♭ (descending).
4. Move on to the 2nd scale degree and repeat.
5. Build a quartal chord off the 2nd scale degree: D-G-C (ascending).
6. Construct a mirror chord, again using C as the reflection point: B♭-F-C.

But when you performed step 6, you used D as the reflection point instead of keeping C the reflection point. If we were to follow your method, then every note would have multiple different "reflections" depending on which chord it's in. This inconsistency strikes me as incorrect.

I believe the correct approach is to use the note C as the reflection point for every quartal chord. This way, every note in the C major scale has a single "reflection." Here are those reflections:

        C → C (both are 0 semitones from C)
        D → B♭ (both are 2 semitones from C)
        E → A♭ (both are 4 semitones from C)
        F → G (both are 5 semitones from C)
        G → F (both are 7 semitones from C)
        A → E♭ (both are 9 semitones from C)
        B → D♭ (both are 11 semitones from C)

Together, these mirrored notes form a descending C phrygian scale. Here's why that's important: every note in the C major scale has a single reflection in the C phrygian scale. If you start with ascending stack fourths in the C major scale, your mirror/output is just descending stacked fourths in the C phrygian scale.

As a result, the mirror you're looking for is simply quartal voicings descending down the C phrygian scale:

        C-F-B (ascending) → C-G-D♭ (descending)
        D-G-C (ascending) → B♭-F-C (descending)
        E-A-D (ascending) → A♭-E♭-B♭ (descending)
        F-B-E (ascending) → G-D♭-A♭ (descending)
        G-C-F (ascending) → F-C-G (descending)
        A-D-G (ascending) → E♭-B♭-F (descending)
        B-E-A (ascending) → D♭-A♭-E♭ (descending)