Chromatic Functional Harmony (Musical Theory of Everything)

Question

When I started studying music theory, I was fascinated by functional harmony. I loved learning why certain chords naturally tend to lead toward certain other chords and why so many chord progressions follow the same patterns.

In my early days of composing (mostly piano, choir and a bit of film scoring), functional harmony drove nearly everything I did. Whenever I'd get stuck (which was most of the time) I'd turn to my functional harmony chart for guidance and it never failed me.

With time I came across more and more techniques that went beyond the boundaries of diatonic functional harmony (secondary functions, tri-tone substitutions, chromatic mediants, axis theory, modal interchange, negative harmony, etc.). With all these extra techniques at my disposal and standard chord functions permanently etched into my brain, my chart eventually became obsolete.

For some reason I have always had a hard time accepting all these other techniques as being outside the bounds of functional harmony. Instead, I see them as extensions, each introducing a new set of functions that can push the piece in new directions. I guess it comes down to the way I think of a chord's function: what role does it play in moving the piece forward?

Recently, it occurred to me that any chord can go to any other chord. Obviously this is physically possible, but what I mean is that every chord has a distinct relationship with every other chord and every change creates motion, be it forward, backward, sideways, up, down or inter-dimensional. In other words, every chord has a function at any given point.

And this (finally) brings me to my actual question:

Is there such a thing as chromatic functional harmony, a musical theory of everything? I have seen some things that come close (axis theory comes to mind), but nothing that truly explains everything.

And, as a followup to that:

If there is no such thing, could it even be theoretically possible? Or are there simply too many combinations to reasonably classify everything in a useful manner?

And lastly:

Would there even be any benefit to something like this? Or are we better off leaving all these additional "functions" where they are, encapsulated in a particular branch of music theory?

Answer 1

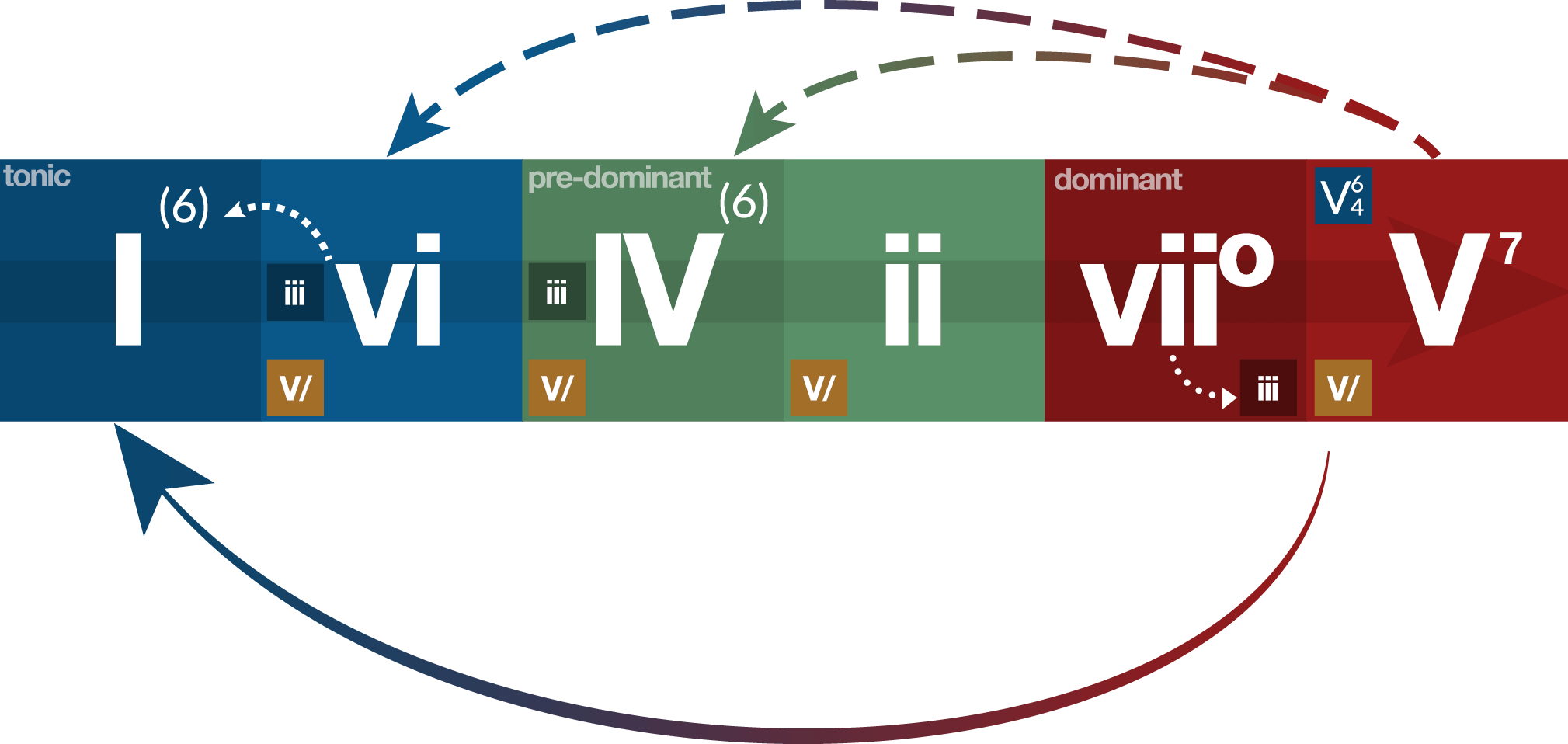
Edit: I tried to construct a meta-model of styles of harmony at the end of this answer.

Functional harmony is a simple narrow "mini-game" that can be played on the larger field of music. If you sit down at a fixed tonic and do the functional harmony thing like it's supposed to be done, so that the listener's sense of tonic is retained, then the functional harmony framework is well suited to reasoning about the things that happen. But there are other kinds of games that can be played, and other kinds of situations where functional harmony isn't the best tool.

Let's take an example of how you might not be properly playing the functional harmony game. If you think about a major key, but then you play only the IV chord for five minutes straight, the listener has no other possibility than suppose that your chord is a I chord. If you then start adding some of what you think are "V" chords, but you keep your "IV" note in the bass as a pedal tone, you might claim that you're playing IV - V/IV, but your listeners will hear it as not a functional harmony at all, but some kind of a modal harmony, maybe a lydian mode of your "IV" note.

Inside the functional harmony game, the sense of tonic is important, and the tonic shouldn't move. If it moves, then AFAIK the movement itself shouldn't be considered a part of functional harmony, even if you start playing the functional harmony game around a different tonic right after the key change. And even if the key-change might seem to utilize the same underlying phenomenon as leverage that works in the V-I motion of functional harmony.

There are many different kinds of games and "player role schematics" that can be overlayed on musical pitches. For example the Barry Harris "sixth diminished" scale system, which could be called a variation of functional harmony. If you use that scale, e.g. C-D-E-F-G-Ab-A-B, and use chords where you take every second note, you get two chords: (1) C-E-G-A and (2) D-F-Ab-B. Chord 1 could be called the tonic because it works as a home chord, and chord 2 could be called the dominant, because it works in that role - but there's no purely "subdominant" role in this game (if we disregard bass inversions). This is very interesting, because you have both the tonic of the "major key" and its relative "minor key" in the same chord C6 or Am7, and both keys' dominants share a chord too, the Bdim7 (and its three aliases).

If you want to see everything that can be done with chromatic notes in a unified and "functional harmony compatible" way, I think you have to think of functional harmony as only one perspective or tool for reasoning about what is where at any given time. If the tonic starts moving around, or if it stays fixed but with different note/chord relationships like in modal harmony, you can still use some of the concepts you've learned from functional harmony, but not completely in their purest forms. For example, and this is just my subjective description, the dorian mode feels almost the same as a regular minor, just with a slight twist. But the lydian mode feels like the harmony is permanently chained down to a IV chord, and no matter how hard it struggles, its feet remain glued to the floor. If just the bass could properly move to the V note and then back home to I! But like I said, this is only my personal subjective feeling, but I'm able to use concepts from functional harmony for thinking about the situation.

My unification of theories

I'll try to formulate my meta-model of harmonic styles and frameworks that I called "mini-games" in the beginning of this answer. (Disclaimer: I haven't studied music in any institution, and I would assume that such theories and models already exist in abundance "out there" in the academic world.) I use a model of harmonic context where each possible perceived situation or position has the following dimensions:

• (T) the tonic
• (C) the set of possible expected chords around the tonic (which is closely related to scales)
• (B) the current balance/imbalance or "leaning" within that set of chords and tonic

At any given time, several descriptions of a position can be simultaneously possible or plausible. For example if you only play a single note, a great number of situational descriptions along the C and B axes feel possible, but the position in the T dimension might feel more certain. So, you actually have a set of (T, C, B) triplets, each with an associated probability.

The harmonic context can move in each of these dimensions, or along the axes. Harmonic movement is a change in the perceived set of probabilities: "how plausible does each positional description feel now". A change in the set of probabilities in itself has a certain feeling and effectiveness to it, and each dimension has its own feeling. A regular pop song chord-change is a change in the B dimension, and a chromatic pop song modulation is a change in the T dimension. (I don't necessarily mean that any of the axes would need to have any specific ordering of positions, though you can probably come up with different distance metrics and definitions of "spatial" ordering inside each of the three dimensions, and such questions have been posted here, like "what's the distance between two chords")

How can we map different harmonic styles to the meta-model?

• Traditional functional harmony assumes that things happen only in the B dimension, and maybe a little bit in the C dimension, but it says almost nothing about the T dimension.
• Modal harmony assumes that inside each mode, the T and C dimensions are kept totally fixed, and even the B dimension is a bit so-so, because there's the risk that leaning too much in any direction is effectively a change in the T or C dimension, making it a different mode.
• Non-modal jazz harmony is like functional harmony, but a bit more relaxed, and it acknowledges the T dimension strongly, meaning that key changes are used in the trick palette.
• In some jazz styles where the tonic seems to move a lot, the change in the probabilities is used as an effect in itself. And some styles might toy especially with having a large set of possible positional interpretations as having a considerably high probability/plausibility. For example, using tritones, dim chords and other symmetric things that easily lend themselves to multiple interpretations.
• In atonal music, probabilities in the T dimension should be low or spread over a large array of positions, meaning that you're not supposed to be able to name any note as the tonic with confidence at any point.
• (insert other harmonic styles here - for example Indian music genres? would they be close to modal styles in terms of this meta-model)

If you consider that the probabilities for each (T, C, B) come from or are affected at least partly by the listener's own internal state and memory, either short-term, long-term and even cultural background, this meta-model could be used to describe a lot of things. For example the relationship between rhythm and harmony, and sense of chord-tone vs. passing tone: if your internal clock sync says it's time for a strong beat/pulse, then the notes you hear get more weight in affecting the probabilities than when you're off-beat.

Could there be a real theory of everything about harmony

To answer the question "could there be a single unified theory like functional harmony but more general" ... I don't think it's possible to describe all possible harmonic styles in the same sentence, so to speak. Each style is complex enough by itself, and follows its own set of rules and assumptions. Even if we could see all the general underlying physical/biological/psychological mechanisms, you would still want to build specialized higher-level abstractions on them, because too much detail is too much. You want to see the forest from the trees and look at things from different perspectives. We have e.g. quantum mechanics, chemistry, biology, psychology and economics as separate fields of science, even though they in some way operate in the same universe. But you can use a meta-model to talk about the differences in perspective and operating domain of each harmonic style.

Answer 2

I can't answer the whole question! But I can suggest that you move on from considering chords as entities but rather look at them as a collection of notes and intervals. You're probably half-way there already in recognising the tritone interval as the driving force within a dominant 7th chord. You'll also have discovered that if that tritone is allowed to resolve (in either direction) it doesn't matter too much what other notes surround it. Now, recognise other tensions between pairs of notes, played together or linearily.

Answer 3

Is there such a thing as chromatic functional harmony, a musical theory of everything?

Even a chromatic theory wouldn't really be a theory of everything, because the chromatic scale only represents 12 pitches out the hundred or more different pitches that a human being can distinguish in each octave.

If we think of what the chromatic scale really is, it's not "non-diatonic", as all diatonic music can (possibly with tuning compromises) be played within the chromatic scale. Rather, it's a kind of "super-diatonic" scale - it allows any mode of the diatonic scale to be played starting from any of the chromatic scale's 12 notes.

For some reason I have always had a hard time accepting all these other techniques as being outside the bounds of functional harmony. Instead, I see them as extensions, each introducing a new set of functions that can push the piece in new directions.

I think that's very reasonable. Perhaps traditional functional harmony can be seen as 'single-dimensional' from a diatonic point of view, in that it functions within a single diatonic scale, while some of these other techniques are multi-dimensional, as the functions move you between different diatonic scales.

If there is no such thing, could it even be theoretically possible?

I guess to me, when you talk about secondary functions, tri-tone substitutions, chromatic mediants, modal interchange... those things do, in a slightly messy way, make up a kind of 'chromatic theory'. Yes, when you talk about 'secondary functions' it does still seem like you're seeing things in diatonic terms - but then the chromatic scale is the twisting, flipping, mutating, transforming cousin of the diatonic.

If you really wanted to make a musical theory of every aspect of harmony, I think you'd start by not initially starting with the idea of scales that restricted you to certain pitches, but by allowing every frequency/pitch, and every timbre - or perhaps even abandoning the idea of 'notes' altogether, and see things simply in terms of changing spectra.