For some two-note harmonies there is indeed
“a scientific basis for why adding a third note
suddenly creates a qualitatively different effect.”
Three effects that sometimes play are role
can be understood after a mention of
combination tones.
1. Timbre (a subtle factor)
2. Intonation (a significant factor)
3. Tangibility (a less scientific factor)
Many two-note harmonies go beyond implying a third note to actually creating an audible illusion of three-note harmony. The result occasionally creates problems that can be masked by a truly-played third note. When things work well, however, is introduced nicely in the weblog A Mind for Madness.
Mathematical Music Theory 3: Combination Tones
[ . . . ]
Composers such as Bach were intimately familiar with this phenomenon. Rather than have it do unexpected things to his compositions, he used it to his advantage. When he wrote two part inventions there would only be two melodies on top of each other, but due to combination tones it sounded much more fleshed out as if many more parts were being played. He would know that in parts where he wanted forward motion he would use unstable forms of intervals and where he wanted resolution he would use the stable forms.
When things go poorly, an actual played third note can rescue a well-intentioned two-note chord gone bad, mainly by overshadowing disruptive effects.
**1. Timbre can be disrupted, subtly.** Combination tones are bland, like featureless sine waves, which can sound intrusive when the original two-note harmony strives for an exquisite timbral blend.
Moreover, these tones will appear with different strengths and blends at different intervals during a two-note harmonic passage, like a third player who keeps changing instruments and styles.
**2. Intonation can ruin a chord.** An illusory third note (combination tone) is sometimes sorely out of tune with the chord it creates. Here is a demonstration that seems to work on every piano, whether acoustic or electronic. Play just the two top notes of each chord and listen for the third (parenthetical) note. The second staff summarizes the resultant chords.
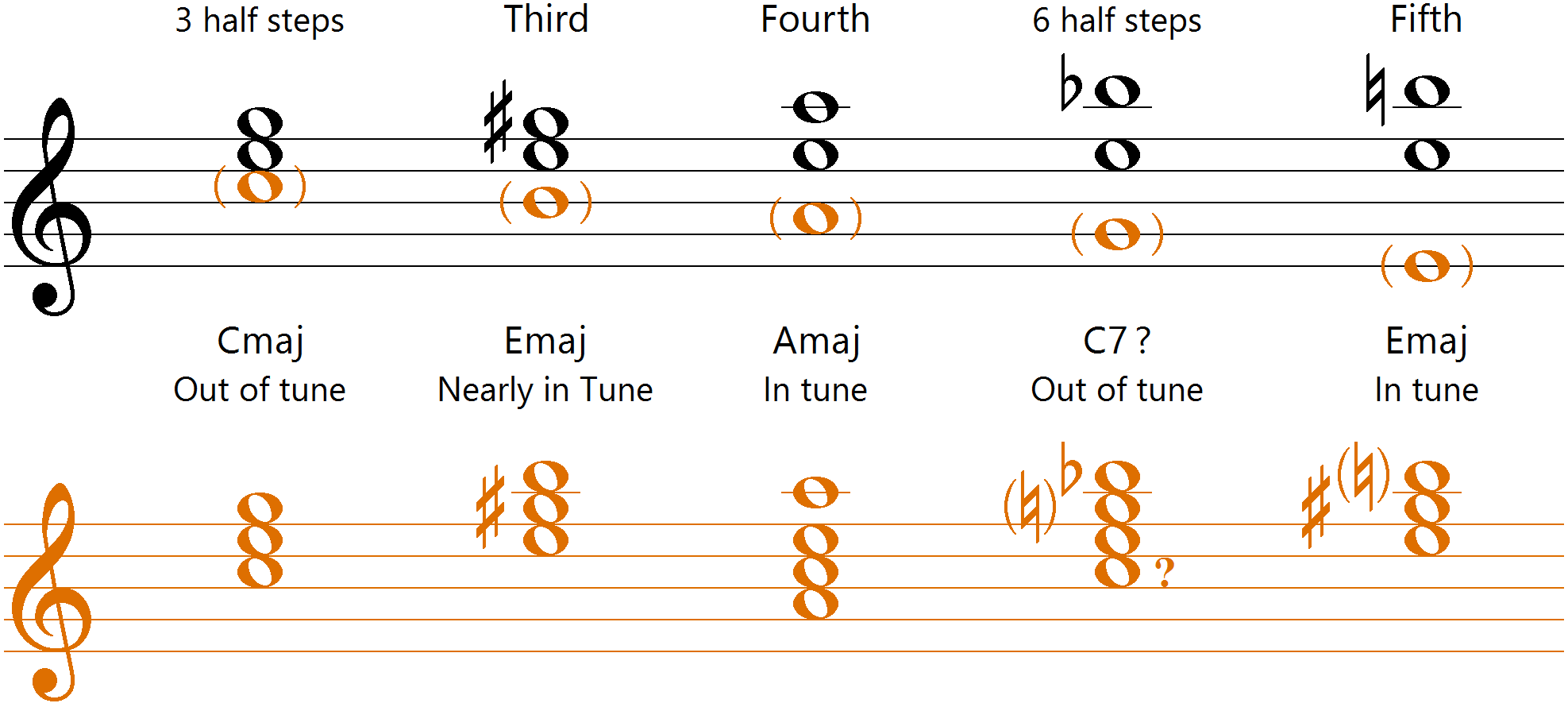
These examples are in a range where combination tones are strong. Exact pitches at play in the first two intervals help understand why they are out of tune to different extents.
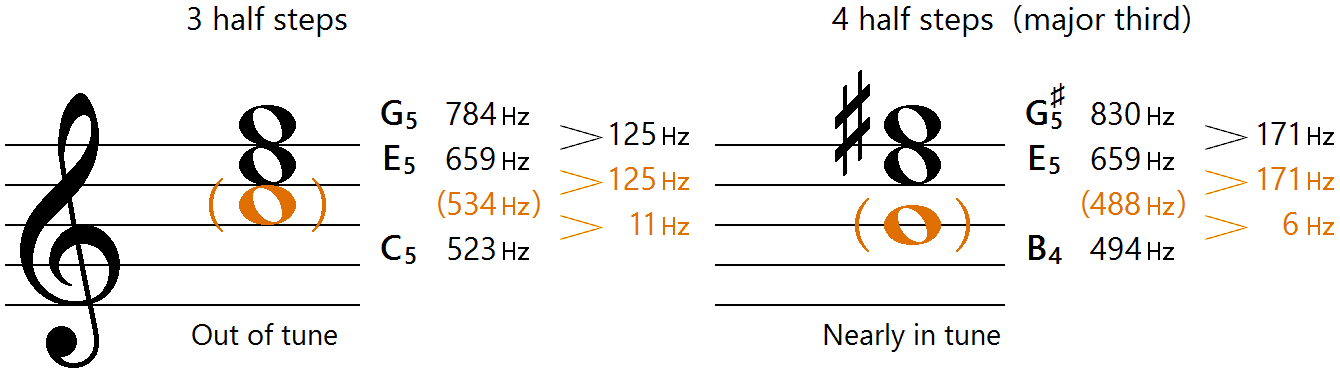
The 3-half-step harmony of E5 at 659 Hz and G5 at 784 Hz creates an illusory note at 534 Hz, separated by the same pitch difference. That new pitch is near enough to C5 at 523 Hz to form a C major C-E-G triad but misses by enough to sound blatantly out of tune.
The 4-half-step harmony of E5 at 659 Hz and G♯5 at 830 Hz works out better. One reason is that the illusory note at 488 Hz is relatively close to the 494 Hz B4 that would be part of an inverted E major B-E-G♯ chord. Another reason is that the 171 Hz pitch difference is relatively close to E3 at 165 Hz, the fundamental note of that chord.
**3. Tangibility, for lack of a better term.** As audible as combination tones can be, they still _feel_ different from played notes. Their incompleteness can unsettle music that is meant to be calm.
This is a minor point, admittedly, but has an irresistible visual analogy. Do you sense faintly-dark blobs where they don’t exist in some corner gaps of this Hermann illusion?
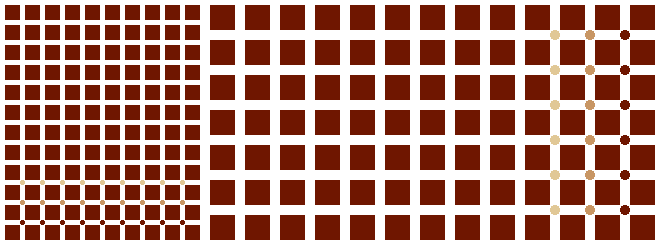
And, before long, aren’t you relieved by places where those blobs actually exist?
**Further Reading**
[Wikipedia: _Combination tone_](https://en.wikipedia.org/wiki/Combination_tone)
[Music SE: _Classical examples of a ‘fifth voice’ or ‘ghost soprano’_](https://music.stackexchange.com/questions/11848/classical-examples-of-a-fifth-voice-or-ghost-soprano)
[Music SE: _Is it possible to create the illusion of a sub-harmonic?_](https://music.stackexchange.com/questions/5824/is-it-possible-to-create-the-illusion-of-a-sub-harmonic)
[Encyclopædia Britannica: _Combination tone_](https://www.britannica.com/science/combination-tone)
[Encyclopædia Britannica: _Noise —The ear as spectrum analyzer_](https://www.britannica.com/science/sound-physics/Noise#ref527375)
[A Mind for Madness: _Mathematical Music Theory 3: Combination Tones_](https://hilbertthm90.wordpress.com/2013/02/12/mathematical-music-theory-3-combination-tones/)
