This answer can hopefully be useful as an additional perspective on top of the existing ones.
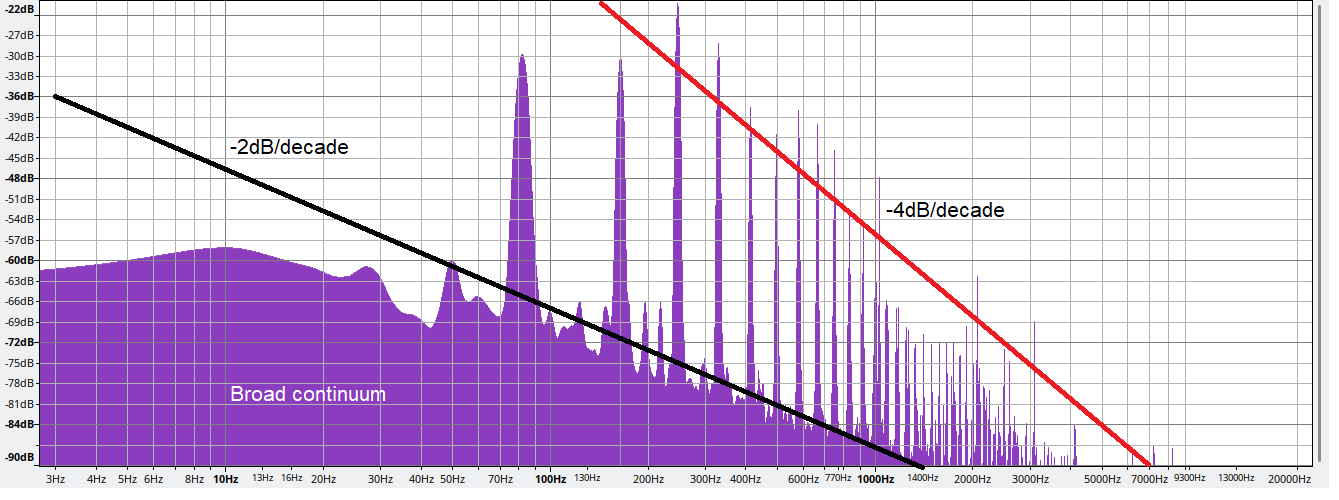
Note that the spectrograph is a plot of (the log of) power output vs frequency (power being energy per unit time). It appears that the fundamental harmonic has been "washed out" into the broad background at low frequency, and perhaps broadened (it's hard to tell because the frequencies are on a logarithmic scale).
There are many reasons why this may be, which could depend on, as others have said, things like where and how you pluck the string, how the other strings and the body of the guitar resonate, and the actual composition and behavior of the string itself. The key point, however, is that the "fundamental frequency" (82 Hz) does, in fact, contain the majority of the power output, but that strings do not actually act like a perfect, ideal string treated in an elementary physics course. There are non-linear corrections to the perfect string behavior which depend on the actual tensile properties of the string (e.g. an ideal string can be modeled as a bunch of springs attached end-to-end with a fixed spring stiffness, but in fact the spring stiffness is a function of frequency and amplitude in a nontrivial way), as well as the resonant effects of the other strings and the body of the instrument, all of which contribute to the "timbre" and rich sound of the instrument.
While for a perfect string one would expect perfect peaks at multiples of the fundamental frequency (delta functions for you math nerds), all of these complicated, non-linear corrections contribute to the broadening of the peaks, and how much each peak broadens depends on the frequency (which we physicists call dispersion). In this case, it appears that a lot of the power in the low-frequency end of the spectrum has been significantly spread out over a broad range, so the low-frequency modes are much more dispersive than the high-frequency ones (this is why you have the broad continuum background at low frequencies which tapers off at higher frequencies). Perhaps others can speculate as to what causes larger dispersion at lower frequencies, but in general, the speed of waves traveling along the string is frequency-dependent, as demonstrated beautifully in this short NPR clip about electromagnetic waves traveling through the atmosphere caused by lightning strikes and detected at the South pole.
I quickly played around with this, and we see something interesting,
As shown in this Physics.SE answer, we would expect that the amplitudes of the harmonics go as the inverse of frequency squared. The power delivered is proportional to the square of the amplitude, and so would go as 1/frequency to the fourth power. I have indicated this by the red line on the spectrograph. Following that red line to lower frequencies would give a rough estimate of the expected height of the fundamental frequency if the string were perfect and there were no resonance effects. However, it appears that the fundamental and to a lesser extent the second harmonic (164 Hz) have lost amplitude and their "spectral weight" has been spread out across a broad range of frequencies at the low end. It's curious that this effect seems to decay as the inverse of frequency squared, indicated by the black line. I don't have an intuitive explanation for this behavior, but I am sure someone could take a stab at that.
One more observation is that the third harmonic of the low E string occurs at about 247 Hz, which is about the same frequency as the D string, this is the third peak. It's possible that the D string is resonating and adding additional intensity to the third peak, but I can't estimate how much we would expect it to add. Similarly the fourth harmonic should resonate with the high E string.
Some more info can be found here.