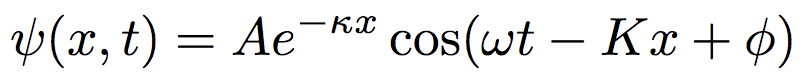When we press a piano key, why does a high pitch note not run for as long as a low pitch note?

Question

When we press the keys on the piano that exist on the ends, one can notice that when we press a high pitch note, it plays for a short bit and then the sounds fades away. However, when we play a low pitch note, it keeps playing for a lot longer i.e we can hear it for much longer. Why don't both the low pitch and high pitch notes run for the same duration?

Answer 1

That's true not only for pianos, but for every instrument where a string is plucked, and the reason is basic physics.

When you hammer a string with a piano key, or pick it with your finger, or with a pick, etc. you impart a certain amount of energy to it, depending on how hard you hit it -- the harder you hit it, the more energy you give it.

Next, you should know that given the same amplitude of vibration, higher frequencies contain more energy than lower frequencies (the string vibrates more often in the same amount of time).

Therefore, if you impart the same energy to two different strings (or to the same string with different lengths), the string that vibrates at a higher frequency will dissipate the energy faster (higher frequency requires more energy) while the same energy on a lower frequency string will dissipate less energy in the same amount of time, and therefore the sound will last longer.

In other words, the total amount of energy dissipated will be the same, but a higher pitched string will dissipate it faster (and therefore last a shorter time) while a lower pitched string will dissipate its energy more slowly and therefore last a longer time.

You can easily test it on a guitar: choose any string, and the first pluck the open string, and let it play. Then fret the string at the highest fret, pluck it with the same force, and note how long that plays. The difference should be pretty obvious.

Answer 2

There is a lot of half answers provided and frankly some of the information is ambiguous, possibly false.

The question itself is not complete enough to elicit an answer. The best I can do is provide a bunch of information that I think is relevant to the discussion and hope that it helps.

Using the simple ideal model for a vibrating string, vibrating plates, etc, the linear damping force is proportional to the velocity of a mass element of the string. When the equations are expressed in the frequency domain this is proportional to the frequency of the wave propagating on the string. From this it is reasonable to conclude that the higher harmonics in a single wave packet will die faster than the fundamental. This is commonly observed in isolated systems. After some time the fundamental is the only noticeable frequency left. The conclusion is also valid comparing fundamentals of different strings.

One has to understand where this relation comes from. There are at least two sources of damping that I can think of for the near ideal string mounted on ideal rigid supports. The first is air resistance of the string moving through the air. This second is internal damping due to the vibrations of the material within the string. In other words energy of the transverse mode (the ideal model) is lost in longitudinal modes in the material and heats it up, increases entropy etc. These are both pretty small but not completely zero.

The first critique of this is that true strings also have stiffness in them and obey higher order differential equations than the ideal string. This does not change the above arguments but contributes to dissonant overtones that are not in the harmonic sequence, fn = n*f1.

Energy is eventually lost from the string to the body of the instrument and eventually to the air as acoustic sound. If this were not possible we would not be able to hear the instrument. This introduces a whole new set of equations, couplings, and physics to consider. The top of a guitar for example would obey a set of equations for stiff plates. They have their own natural harmonics that may or may not be aligned with those of the strings. Part of Luthier's art is optimizing this. So depending on the quality of the instrument and its condition some notes may get amplified more than others. This is a very common occurrence with acoustic stringed instruments and something we test for when purchasing an expensive instrument. You check for buzzing, dead spots, and RESONANCE. You want resonance to some degree as that adds to the sound but you don't want anomalous resonance which might show up as Bb4 is always 3dB louder than any other note (just a silly example but not impossible).

This brings me to an important point. That the rest of the instrument will vibrate in sympathetic resonance to the note being played and its harmonics.

The harmonic content in the string depends on the attack. Not all strings are the same. In fact one could argue that this is the most important part of sound and the hardest part of learning an instrument, learning proper attack and for guitar learning variety of attacks. Each attack produces a completely different "tone". This makes the guitar a great mimic and has a reputation for versatility. In contrast your piano hammers are fixed. You can control amplitude (strength of attack) and with pedals you can control sustain but you cannot control the initial attack profile of the string(s). Keep in mind that each "key" strikes several strings not just one.

Now typically (but not always) the fundamental is the strongest note, has the highest amplitude or volume among the spectrum of the string. And linear systems do NOT excite sub-harmonics. They don't even excite harmonics for that matter. The other strings will vibrate in sympathetic resonance to the string you play but only if the harmonics of the string are present in the one you played. And they will only vibrate at the frequency of that harmonic. A caveat to this is that coupling with other parts of the instrument could cause coupling between different modes due to a non-linearity, perhaps a joint in the wood etc. thus causing coupling between harmonics. But for the most part the linear model works well. For example if I play the high E string on my guitar, and assuming I attack it so that only the fundamental is present (close to possible if you use your thumb at the 12th fret) then that E will cause the following resonances in the other strings, n = 4 on the low E string, n = 3 of the A string, nothing noticeable on the other strings even though E might be close to a harmonic for some. The presence of these extra notes will add to the volume of the note plucked. As for sustain, you might think that since these are all the same frequency that they would all suffer the same damping. This is true. But you are judging the "decay" of the note by whether you hear it or not and the added amplitude means that the sound will not drop below detection threshold for a longer time. In contrast if the low E string is excited the same way it will NOT cause sympathetic resonance in the other strings. It will be less audible than its higher pitch counterpart.

This brings us to another point. If you are using your ear to make this judgement I don't trust any of it. The human ear is highly non linear in both amplitude and frequency. Our ears create harmonics from the input. This means that even if the higher harmonics are NOT present in sound YOUR EAR WILL HEAR THEM. There is no way that the physics of the instrument can change this. The ear+brain system hears higher frequencies better than lower frequencies to some extent, possibly related to the last point. Bass and treble notes played at the same driving force will be judged as having different volume by listeners. For a Bass note at 100Hz and a high note at 2000Hz both played pianissimo the Bass note may not be heard by anyone. So any claim made about hearing low pitch notes for a longer time is suspicious without more information.

I can say that on the guitar it is simply not true that the higher pitch notes die quicker than the lower pitch notes. Of course there are too many variables to make any answer to this question complete and absolute. If you are really interested in the behavior of the musical instrument and your own ear for that matter, each variable needs to be isolated and the cause and effect relationship to other variables quantified before trying to make blanket statements about "the instrument". I'd suggest looking at a text like "Physics and the Sound of Music" by Rigden or something non mathematical (assuming you are a musician and not a scientist/engineer/etc) by Fletcher and Rossing.

EDIT:

As a final note I will say this. The hammer placement on a piano means that you will likely excite higher pitch harmonics with each note. This is the opposite situation as my guitar example where I imaging thumbing it on center (like Wes Montgomery). In such cases the lower strings will have a chance to excite many more other strings in the harp, each at the higher harmonic. Again using the guitar example, if I play the low E string but pick it near the bridge I will excite the open string B string (n = 3) and the open high E (n = 4). These will vibrate in their fundamental mode of vibration as those frequencies match the higher harmonics of the low E. NOTE: plucking near the bridge is critical for this to work well. So it is possible that lower stings in the piano have several octaves of strings helping to support the harmonics. But again, as the string motion dies I question if it is the low pitch fundamental that you hear or the ringing of all the harmonics. It would be natural to associate this ringing with the string you hit but that isn't necessarily true. It may be all the others. This in no way contradicts the previous example but serves to illustrate the complexity of the instrument and that with the correct conditions either phenomenon can be observed.

Answer 3

This is a really interesting and complicated question in the physical simulation of string dynamics.

Actually, it’s not entirely true that high pitch notes run shorter. There is a tendency for higher order partials (inharmonic overtones) to decay faster (run shorter). But due to the complexities of piano tuning and string coupling, it is not true that if you play each note on a piano one after another, the next higher note will always decay faster. Also, you will find that each partial within a particular note might decay in a different manner, and this pattern of decay varies per note.

Tackling the simpler part of the answer, one of the reasons there’s a tendency for higher order partials to decay faster can be seen in a simplified model of string vibrations.

The wave equation of a simple oscillating string, with decay, can be modeled with the formula.

The gist of the formula is: the bit on the left, ψ(x,t), determines the displacement of the string at some point x and some time t. The goal of this wave equation is to determine where every point will be at every time, which is the same as knowing vibration frequency and amplitude. The stuff inside the cosine on the right can be ignored for this discussion. But the key important thing is the exponential e^-κx. κ is proportional to the frequency of the wave. Which means as frequency goes up, κ goes up, which means the exponent shrinks, and so the physical displacement of the string from rest is decreased. In other words, there’s decay dependent on frequency.

This isn’t the full story, but does indicate that high frequencies lose energy to the air faster. There are other sources of energy decay though, like loss in the sound board of the instrument.

Other complexities arise, like the coupling between strings. Take an A4, which has three strings. If all three strings are tuned to the exact same frequency, the decay rate will be three times faster than a single string. However in practice the strings are tuned very slightly differently, creating beats in higher order partials. If one partial decays faster than others, because of coupling, it transfers energy into the others. Thus the note is sustained much longer. As you move along the piano, from lowest to highest, some notes have one string, some two and the majority have three. There will be kinks in the decay pattern as you transition across these regions.

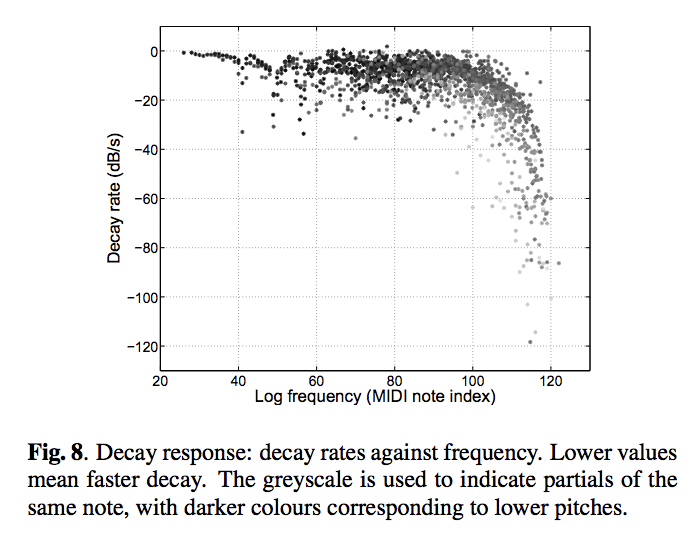
The diagram below comes from the paper http://matthiasmauch.de/_pdf/cheng2015modelling.pdf and does a good job explaining how piano notes decay depending on their frequencies.

To explain the graph, the x-axis is frequency (given in midi note index. To give you a sense of conversion, MIDI 57 = A3 (220Hz) and MIDI 69 = A4 (440hz)). The lower you go on the y-axis, the faster the decay.

Clearly, as you move right the data points start moving down (the higher the frequency the faster the decay). But there’s not a smooth curve; there’s a cloud of points. So, if you take any one point on the x-axis, let’s say MIDI 69. There is a spread of y values, i.e. different decay rates. There’s also a spread of grayscale, which means that this frequency will decay at lots of different rates depending on the how high order a partial it is!

I know it’s not a terribly satisfactory answer, but the underlying physics is sufficiently complex that a satisfactory answer doesn’t quite exist. The gist is: usually higher frequencies decay faster, but not always, and there are complicated reasons why!

Answer 4

The higher the string, the shorter and thinner. The lower the string, the longer and thicker. The lower strings have more mass and do not let go of the vibrations as quickly as the higher strings. Additionally, the lower strings have more harmonics and more opportunity to resonate with other strings in the piano, which adds to the sustain. If you need more detail, you will probably need to find it in a physics text.

Answer 5

Aside from the increasing momentum of lower pitch strings, note that the damping force is practically the same for all strings during free vibration. So the loss rate of energy is the same for any string. This makes lower pitch strings take longer time to consume their energy.

One can design a special instrument that gradually increases the damping force as the note goes lower so that the sustain times become equal. However in this special case, still the damping force of the resonating amplifier body (for example the wood board of a guitar or piano, as well as the room around the instrument) would stay the same and provide slightly longer sustain for lower notes.

You can test this phenomenon on any string instrument. Just play a bass note and then stop it using your hands/bow, and repeat the same for a high note. You will hear that the bass note resonating longer on the board.

Also note that pianos have bigger hammers and dampers for the lower notes for the same reason. You need to generate more energy and then consume it back.

Another example is, on a piano you can hear low notes sustaining longer than high notes when you lift your finger from the key.

Answer 6

Let’s consider a wire strung between two posts. After you hit it with a hammer two waves pulses propagate up and down the string, one in each direction. They hit the end posts, bounce in the other direction, and so on; two pulses racing back and forth along the length of the string.

The fundamental frequency, ie the pitch of the string, is the inverse of of the round trip time.

First, consider the case where the internal friction of the string is very low. Then the losses occur when the pluses hit the end posts. For higher frequency vibrations, this happens more rapidly. Assuming that the same fraction of the wave energy is lost from the string (and transferred to the sound board) each time, you’d expect the sound to dissipate more rapidly for higher frequencies.

Similar considerations apply when thinking about internal losses — a given short segment of the string flexes and then flattens as the pulse moves through it. And again, if each flex dissipates some energy, then more energy is lost per unit time for higher frequency strings. But this gets confounded by the fact that there are more such short segments in a longer string. A naive application of this logic leads one to conclude that this might result in a frequency-independent term of the losses. (From there you can think about whether the internal losses depend on the rate of change of the shape...)

So, to first order, keeping everything other than the length of the string fixed, you’d expect that higher pitched strings would lose energy more rapidly than lower pitched ones.

Of course, in a real piano not everything else is held fixed, and then when you start to consider the fact that the perception of loudness depends on frequency, then things getting more complicated fast.

Answer 7

I'm guessing, but because it vibrates at a higher frequency (being a higher pitch) the energy put into the string by striking it with the key, simply is used quicker. All keys, or strings get the same amount of energy when hit with a key (artificially, I get into it next paragraph), thus a quicker vibration vibrates and moves more in a given period of time, releasing energy quicker, thus running out.

The string size differs, the smaller strings being higher pitch, allowing and creating it to be moved or vibrate more (quickly). Allowing the large stings to store more energy and release it slower, in a pitch with a higher frequency (or less vibration, or movement back and forth in a given time). Small things take less energy to move, so the strings move more, and because of the movement being quicker, or a faster pace all energy dissipates before that of the large/wide/thick low notes. You will notice either; thicker strings should be harder to move or take more energy, or that Pianos have 'hammers' that vary in size, the lower the note the more large the 'hammer'. Those two things actually balance each other out. Large hammers are used to strike the thick strings because to get the volume/amplification of the high strings you need more energy. This in a way makes up for the fact that the strings are larger, leaving you with the simple fact that less mass takes less energy, thus the energy is used much faster, in the form of vibrations (at a quicker rate).

The size doesn't actually matter, what matters is how fast the sting is vibrating, that is what creates the sound waves. If you concentrated more energy into a single point and hit the thicker stings, they would vibrate more violently than less energy. The more vibration the higher the frequency, witch equals higher pitch.

You don't have to really think about the string size in the matter of how much energy it takes to move, because the hammer size does that. Look at it of more the size, or the smaller object is easier to move, so moves faster, running out of energy first.(don't worry about energy to actually move it, as the hammer increases with string size, artificially making the amount of energy needed to move the string is the same, however not the same amount of time)

Sorry if it's hard to understand, it makes clear sense in my mind, kind of hard, and a bit abstract to explain it, and the way I think about it.

Hope that is a quick simple to the point answer.