Tritone or Minor 2nd as the most dissonant interval? [closed]

Question

I'm trying to establish which interval is more dissonant, the tritone or the minor 2nd. Both are obviously very dissonant intervals.

Math seems to prove that a minor 2nd should sound more consonant due to the ratio of 16:15 vs. the tritone ratio of 45:32.

But when we define consonance as "the time 2 sine waves take to both resolve at 0 during the same time", then the minor 2nd is more consonant due to its earlier "resolution" compared to the tritone.

Here is is a m2 and tt played in equal temperament as sine waves. Please compare both intervals to decide which one you feel is more dissonant.

Based on the above do you think that this definition of consonance makes sense and that the minor 2nd is actually more dissonant than the tritone?

Answer 1

TLDR: The minor second will be heard by most people as a lot more dissonant.

Longer version -

Subjective dissonance can stem from (at least) two causes:

• Two tones having a non-simple ratio, such the ear doesn't tend towards trying to hear them as a 'single sound'
• Two tones being within the critical band, such that the ear has difficulty distinguishing the individual frequencies, and instead hears a beat frequency

The minor second does arguably have a simpler ratio - but it's within the critical band (which is about the width of a minor third), and so is very dissonant for that reason.

This diagram (from http://sethares.engr.wisc.edu/consemi.html) shows results from an experiment by Plomp and Levelt, how the sound of two simultaneous sine waves was judged to become less dissonant once their pitch difference is beyond this critical band:

You will also see on that page that the same researchers found the dissonance curve for more natural musical tones (including overtones) was different...

...the explanation being that the overall roughness heard was a combination of the roughness caused by all combinations of the component sine waves. (Of course different instruments have different timbres with different sinewave components, which is why different harmonies work differently on different instruments).

If I'm allowed to add my own perspective - I do think I tend to hear sinewave difference dissonance a little more like the 'natural tones' result - in other words, My 'critical band' response is as Plomp and Levelt found, but I also 'appreciate' or 'recognise' a simple or near-simple ratio a little more than Plomp and Levelt found their subjects to when it comes to sine waves with differences beyond the critical band.

Answer 2

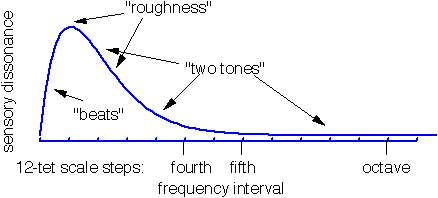
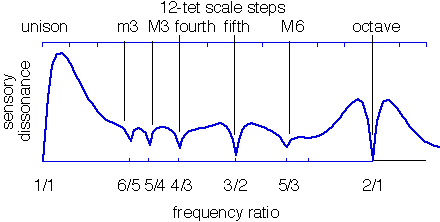
There is no doubt that simple integer ratios between two frequencies produce consonant harmonies. The reverse, however, is not true. If it were, equal temperament would be completely unusable, since, as @badjohn points out, the ratio of every interval in equal temperament (except the unison and its octaves) is an irrational number.

But I'm not so quick to dismiss mathematics as an objective measure of consonance and dissonance. It's just that looking no further than the complexity of an interval's ratio is grossly oversimplifying the phenomenon.

For one thing, the simpler the ratio, the easier it is to hear dissonance when two frequencies are off. Your ear hears sharp or flat unisons more easily than sharp or flat octaves, and, in turn, it hears those more acutely than sharp or flat fifths. Were it not for this fact, equal temperament could just as easily have expanded the octave as narrowed the fifth. (As it happens, the span of 12 fifths is reduced to 7 octaves, not the other way around.)

It's apparent from the graphs in the answer by @topo morto, too. Perhaps it's that we don't hear a minor second as a "simple" 16:15 ratio but as a really, really off 1:1.

That said, slightly off intervals which are very complex but nevertheless approach simple ratios (like a unison) are actually much more interesting to the ear than perfect harmonies. Consider choirs and 12-string guitars, for example. They produce the chorus effect naturally because the constituent sounds are not in perfect harmony. Anyone who's ever messed around with a synthesizer knows that slightly detuning the oscillators creates a richer, more interesting and pleasing sound.

Besides the factors of interval span and timbre, another consideration is the pitches at which intervals are demonstrated. For example, an A7 chord rooted at A1 on a piano sounds awful. The parallel chord two octaves higher is quite nice, despite containing a tritone between the C♯ and the G.

Finally, context also plays into the perception of consonance and dissonance. For me personally, the minor seconds in the Angry Birds theme, while dissonant, are delightfully playful. (The bit with the minor seconds starts around 0:20.) On the other hand, the minor second in your example is torturous. For me, it's clearly the more dissonant interval.

Answer 3

Mathematical analysis of the frequencies is attractive but it can be easily taken too far. If the simplicity of the ratios was the only factor then any interval on a well tempered instrument other than whole octaves would be maximally dissonant as the ratio would be irrational.

Even if you ignore this factor there are oddities. Play a perfect fifth (e.g. C and G). Now play a major third (C and E) and a minor third (C and E♭). Which sounds nicer? This is subjective of course but many would say the thirds. It is for common for two parts to be a third apart but rare for them to be a fifth apart.

Play a tritone (e.g. C and G♭) and most would agree that it is dissonant but add a couple more notes to make a typical diminished chord (C, Eb, G♭, A/B♭♭) and it sounds much less dissonant.

What does and does not sound nice cannot be explained by simple mathematics.

Note that is not anti-maths bias, I am more qualified in maths than music. In fact, I would say that it is my knowledge of maths that allows me to see the limitations of this idea.

Answer 4

You're using a classical but troubled definition of consonance in which an interval whose fundamental frequencies are in a simpler ratio (smaller integers) is more consonant. This doesn't match practice: your minor second with a ratio of 16:15 will likely be perceived as more dissonant than a tempered fifth with a ratio in the ballpark of 1.499:1

With no musical context, I find a minor second more dissonant. Perhaps others feel differently. However, which is more dissonant in the music at hand depends on the music. More factors enter into play: dynamic level, harmonic function. The terms consonance and dissonance are overloaded.