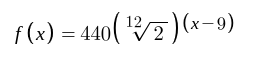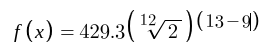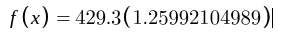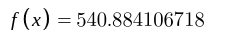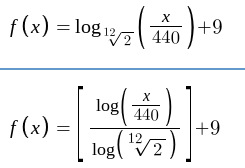Suppose you are trying to reproduce an A major scale from the early 18th or 19th century, when A4 was perhaps tuned to 429.3Hz. What is the correct frequency (in Hz) for the next C# up (assuming we want an equal tempered scale)?
I need a little help with figuring what the sequence is in this question and how to figure out how to calculate the whole whole half whole set up.
The question is asking you to calculate the frequency of C# based on an equal-tempered scale and a frequency for A of 429.3Hz.
The portion of the homework assignment that you deleted between the first and second versions of your questions gives an example of a similar calculation, using a different value for the frequency of A and seeking to calculate the frequency of a different note.
So you should, as the problem suggests, calculate the pitch of B when A is 440 Hz to confirm that you arrive at the correct answer. Then you should be fairly confident that you can calculate B when A is 429.3 Hz.
But the problem, of course, is to calculate C#, not B. So you also need to figure out what is different about that calculation. The difference lies here:
the pitch of B4 can be found by multiplying 440 by the square of the twelfth root of two, since B is two semitones above A and each semitone raises the pitch by the 12th root of 2.
C# is not two semitones above A, so the frequency of C# is not to be found by multiplying by the square of the 12th root of 2. Part of your task is to find the actual factor you need to use instead.
In 12-tone Equal Temperament (the modern ubiquitous tuning system for most instruments), the mathematical formula for the frequency of any note is:
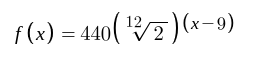
Where f(x) is the frequency of the note in Hertz, and x is the number of semitones your note is above Middle C (C4).
This is because an octave is defined to be exactly twice the frequency of its starting note, and there are twelve semitones in an octave. Don't let all the fancy math symbols confuse you; this is basically just saying that you multiply 440 by the twelfth root of two once for every half-step higher up you go. And of course, the (x-9) is just to get everything relative to middle C instead of A4 (for the musicians), which is defined to be 440Hz.
In your example, however, A4 is defined to be 429.3Hz. This means we'll substitute into our formula like this:
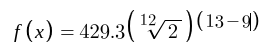
Notice since we started with A4=429.3Hz, the formula has changed. Also, C♯5 (the C♯ above A4) is 13 semitones above middle C, and obviously 13-9 simplifies to 4 (or you could reason that C♯5 is 4 semitones above A4. Same result!).
Simplifying,
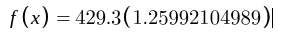
and we find that
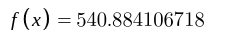Et voilà, your note has a frequency of 540.88Hz.
This is the pure mathematical way of doing this kind of problem. There are probably other ways to do this, but this way is pretty cool and it does make a lot of sense. Also, that formula in the beginning is the general case formula, and its inverse is useful to go from a known frequency to a note:
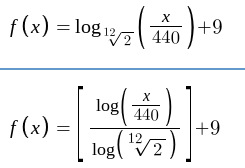
Where f(x) is the number of semitones above middle C, and x is the frequency in Hertz. The two formulae above are equivalent.
Note: You might end up with a decimal output, whic is okay, since not all frequencies correcpond exactly to a note in 12-TET. So if you ended up with, say, 16.21 as your output from those functions, you know your note is slightly higher than D5.
