I enjoy jamming over Sway by the Stones. The final solo is C minor pentatonic mainly played over two chords, C major and Bb major. While I understand why you would use a minor pentatonic on a major chord I was interested what the Bb chords function is in the end solo as it alternates with the C chord? Also where does it come from if we are playing in C major?
If the C where minor then it makes sense as the VII chord but in major?
Cheers!
...what the Bb chords function is in the end solo as it alternates with the C chord?
If it is just going like C Bb C Bb C Bb... then looking for function doesn't make sense. It's static harmonically. You can just call this kind of two chord alternating a vamp. Function in the traditional sense is about predominant to dominant to tonic harmonic progression and cadences. Rock music often doesn't work that way. It's OK to abandon functional analysis when the music is working from that playbook.
...where does it [Bb major chord] come from if we are playing in C major?
You can call that a borrowed bVII chord. Borrowed from the parallel minor. Or, you can think of it as the triad build on the lowered ^7 scale degree in C major. That change to ^7 turns the C major scale into C Mixolydian mode. This is a kind of Mixolydian 'coloring' that is very common in rock music.
EDIT
@Ramillies raised an interesting point: why is Mixolydian mode popular in rock music?
I offer up these two explanations. Really it's just two sides of the same coin:
^7 scale degree) and technically the music will not be able to produce an authentic cadence. That removes a major musical factor that could disrupt the continuous flow of a groove.IV to I. I say "so-called" because rock music doesn't normally cadence - it doesn't stop and end phrases in the classical sense - IV to I would be better called a plagal progression but that's a separate matter. Anyway, this progression is hugely characteristic of rock music. It gives a strong sense of tonality without the strong sense of cadence implied by V to I. Add to this the fact that the lowered ^7 degree over the tonic chord makes a V7/IV chord - the dominanat seventh of IV - and we can see how shifting to the Mixolydian mode pushes the music toward the IV chord.Basically, lowering the ^7 degree moves the tonality away from authentic cadences with V to I and instead toward plagal progressions IV to I.
Keep in mind, when I say cadence and end this isn't the same as rest and silence. There are plenty of breaks to be heard in rock music. Those aren't cadences.
None of the above means that rock music doesn't use V to I. That's very common too. This is just my attempt to 'connect the dots' about several rock music characteristics: groove, Mixolydian, and IV to I.
It's flirting with things and keeping you in suspension. "Is this major or minor" and "where is the tonic" are the questions you're supposed to have.
There are many ways to look at it, but here are two of them:
In my opinion, a combination of (1/2) and (2/2) is the most natural way to look at what's happening, and what could be happening, i.e. what possibilities it gives you as a soloist. The switching between minor and major is a "thing" in rock/blues/jazz. The set of "correct" notes is not very rigid - you can overlay e.g. C minor pentatonic, G minor pentatonic, C blues, and many other things on top of the backing chords, and it would all be equally fine. The song should encourage you to jazz it up and play around with the harmony.
I wouldn't try to explain someone that what's happening is C mixolydian mode, and by being able to create the mixolydian sound you could reproduce the things you hear on the actual recordings of the song. You can't, mixolydian is not enough.
If you want to experiment how the "fluidly moving between major and minor" way of looking at it works in practice, try the following.
Try replacing the repeating C - Bb pattern with C - Gm. Does it work the same? Can you still flirt as freely with the E i.e. "it's C major", on the Gm chords? How about if you replace the C majors with minors, so Cm - Gm - what happens now? How about Cm - Bb? I recommend trying out different harmonic options in practice and soloing over those, to get to know first hand how the backing chord notes work together with your soloing notes. If you're brave enough, try ending the C - Bb vamp on a final Ab major chord - what happens to your harmonic interpretations? How about if you play Am as the final chord?
I cannot stress enough the importance of experimentation. You learn to actually know things only by interacting with them. You can learn grammar and memorize words as much as you like, but when you actually have to get your business done by using the language, it's worth more than a thousand grammar lessons.
It's quite simple — it is borrowed from the C mixolydian mode. Which is a pretty awful name, but the concept is very simple.
For start, let's take the C major scale. Here it is, through two octaves:
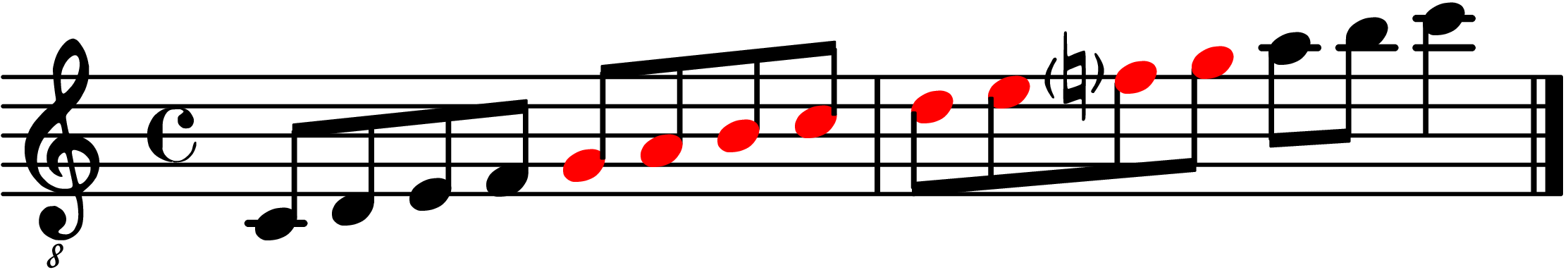And now, play all the same notes, just start with a G. You will get a "scale" that is highlighted in red. You can see that it's pretty much a G major scale, only with the 7th tone lowered. This is called the G mixolydian mode. (The name comes from the Ancient Greece. This (and the other mode names) were later taken over by the Roman Catholic Church, which used them for quite some different things, so the name has absolutely no meaning. It's just a millenium long tradition.)
Of course, we can transpose this around easily by noticing that the mixolydian mode is just a major scale with the 7th degree lowered. So a C mixolydian would look like this (look at the first bar):
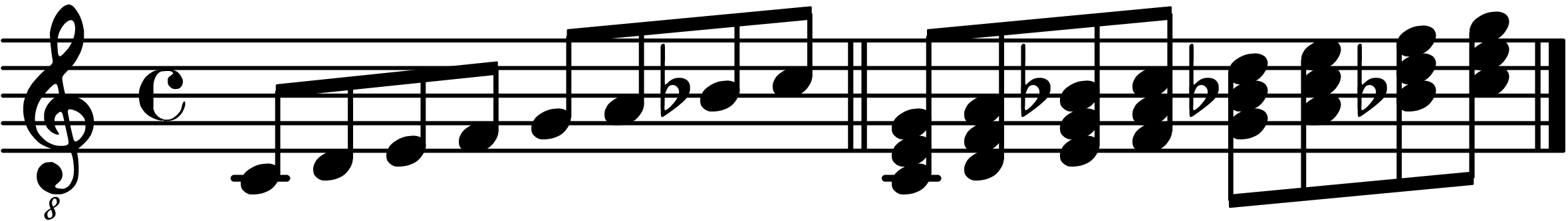
Of course, you can stack the tones just as if you would with your normal C major scale, and generate some basic triads that go well with the mode. In the C major scale, you would get these triads: C major, D minor, E minor, F major, G major and B diminished. When we flatten the 7th tone, you get the chords shown in the second bar above, namely C major, D minor, E diminished, F major, G minor, B flat major.
So a couple of chords changed, and from among the changes, your B flat major chord appears.
Now of course this doesn't explain why is it such a good idea to use it in a song, and that's always hard to tell, because the only real reason is just "it sounds good". However, I can at least try to give some theoretic reasons:
It's kinda the major scale that everybody hears 1000 times a day, but not quite. It adds a bit of novelty while not being anything totally weird.
To go deeper: in the major scale, the 7th tone is only a semitone from the tonic (the next tone). For instance, in C major, the 7th tone is B. For some reasons, this B can pull quite strongly towards the tonic (C). If you flatten it, it doesn't pull nearly as much, giving a different, maybe more "calm" sound.
Also Michael is very correct in saying that there are other possibilities of explaining the origins of this chord. That's always the case with the music theory; it's never set in stone. It's not math. With just two chords alternating, it's not possible to prefer any one explanation above the others, because the chords have pretty much zero harmonic functions. So this is just one view on it.
Having listened to the song,
The final solo is C minor pentatonic
To me it's C blues, with all the bends and blue notes that implies. The blues scale is in some places better expressed in terms of ranges though which notes can be bent, rather than specific notes.
Also where does it come from if we are playing in C major?
Again, I wouldn't describe it as C major. There are enough places where an Eb is hit to my ears to take it away from being major, and again, give it a feel that's best described as blues. There's a type of blues-rock harmony that is based around basing power chords on the 1, b3, 4, 5, and 7 degrees of the scale; that style is quite similar to what we have here (and can also be expressed in terms of the Mixolydian, as others have mentioned). There are E naturals in evidence too, but that is entirely in keeping with the major/minor ambiguity of the blues scale.
