Thicker picks (tend to) remain in contact with the string longer. The impulse provided to the string is of longer duration. A longer duration pulse imparts more lower frequency and less higher frequency content.
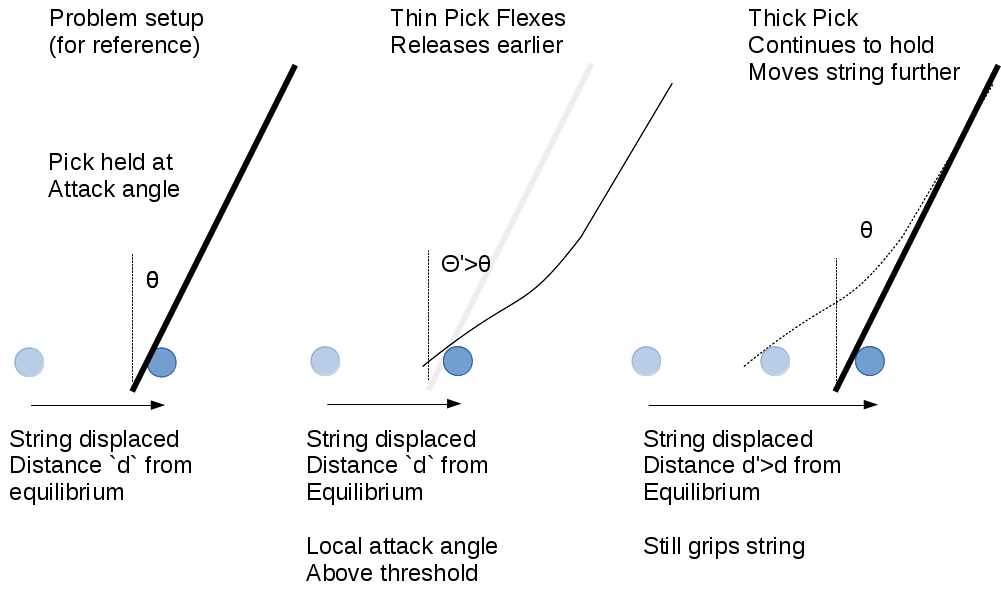
Imagine the thick pick, at an angle, coming in to hit the string. It strikes the string, which starts to move along with the pick, but the pick itself is not deformed much initially. Driven by the hand, the pick continues to press the string downward. Eventually the downward motion of the pick, along with changes in its attack angle (even if the pick itself is very stiff, there is some play just in terms of your finger tips) get to the point where the string is "released" from the pick and starts to vibrate freely. This release occurs because while you are pushing the string further down, the string is pushing back more and more; eventually the tension in the string, along with the changes in the attack angle of the pick, get to the point where the string starts to slip and is released. The amount of time from the initial impact of the pick on the string to the time that the string is released bound/defines the time span of the impulse that is provided to the string.
The interaction of a thinner pick is the same, except that the pick itself now flexes; this means that the string will release from the pick sooner: the pick is flexing more and less able to resist the back pressure of the string.
Though this glosses over some of the details string-pick interactions, we can look at when the string will start to slip in terms of the following diagram
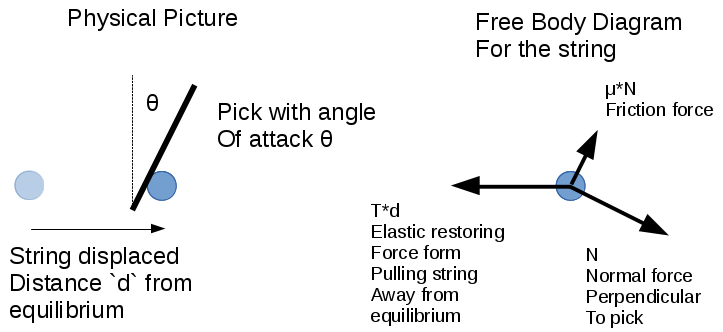
Assume is already in contact with the string and displaced it a distance d from its resting position. Also assume that the pick, at the point of contact with the string is at an angle $\theta$ from the normal to the plane spanned by the vectors along the length of the string and along the picks direction of motion. In order for this point of the string to continue moving with the pick (I'm assuming that the picks speed is kept constant by the guitarist) the net forces need to be zero (no acceleration). Three forces are in play: the restoring force due to the tension in the string, the normal force, and the static friction between the pick and string. We only need to consider the vertical components of the force in the diagram above, which yields the equation:
$\mu N \sin \theta - N \cos \theta =0; solving for the static coefficient
of friction yields $\mu=\tan \theta$. Given that there is a maximum value for the static coefficient of friction (determined by the material composition and surface structure of the pick and string) above which the string will start to slip along the pick, there is also a critical angle of attack. Getting back to picks, for a thick (stiff) pick, the attack angle only changes from flection in the finger tips; for a thin (flexible) pick, the pick itself flexes, increasing the attack angle. Thus the thin (flexible) pick releases sooner (everything else held fixed) because it "bends to get out of the way", as in the following, exaggerated for effect, picture.
This is essentially a qualitative version of the model described in "Synthesis of Guitar By Digital Waveguides: Modeling The Plectrum in
the Physical Interaction of the Player with the Instrument", F. Germain and G. Evangelista, 2009
The state of the string when it releases from the pick sets the initial conditions for the string's vibration. The model I have in mind involves
- The string is at rest, and straight.
- The pick is moving downward at some rate dictated by the hand.
- The pick first strikes the string.
- Due to friction, the string moves along with the pick (and the hand) at that rate. I've idealized the area of contact to be a point.
- That point on the string continues to move downward at the same rate as
the pick until a the static friction threshold is reached, and the string is released.
- After this point the string freely vibrates.
At this time, the pick has pushed down at one point in the string. The "height" of the displacement is (hand-speed)*(grip time) -- while the pick "grips" the string, it is moving with it (and with your hand). The deformation caused by the pick spreads out from the pick at the wave speed in the string. The half-width of the tent along the string is (wave-speed-in-string)*(grip-time). Thus the width of the pulse in the string, and thus its spectral content is affected by the amount of grip time. Points on the string further away cannot yet be affected since disturbances travel through the string at the string's wave speed.
We can run through the numbers for an A string, fundamental frequency 110Hz, length 650mm (rounded for convenience). I assume that the pick is moving downward with the hand at a rate of 1m/s. The pick grabs the string, at a point 150mm from the bridge, and causes that point of the string to move downward at 1m/s. I've assume a "long" grip lasts 1/1000th of a second and short grip of half that. That is, in the "long" case the time interval from the point at which the pick first hits the string to the point at which the string is released to freely vibrate is 1/1000th of a second.
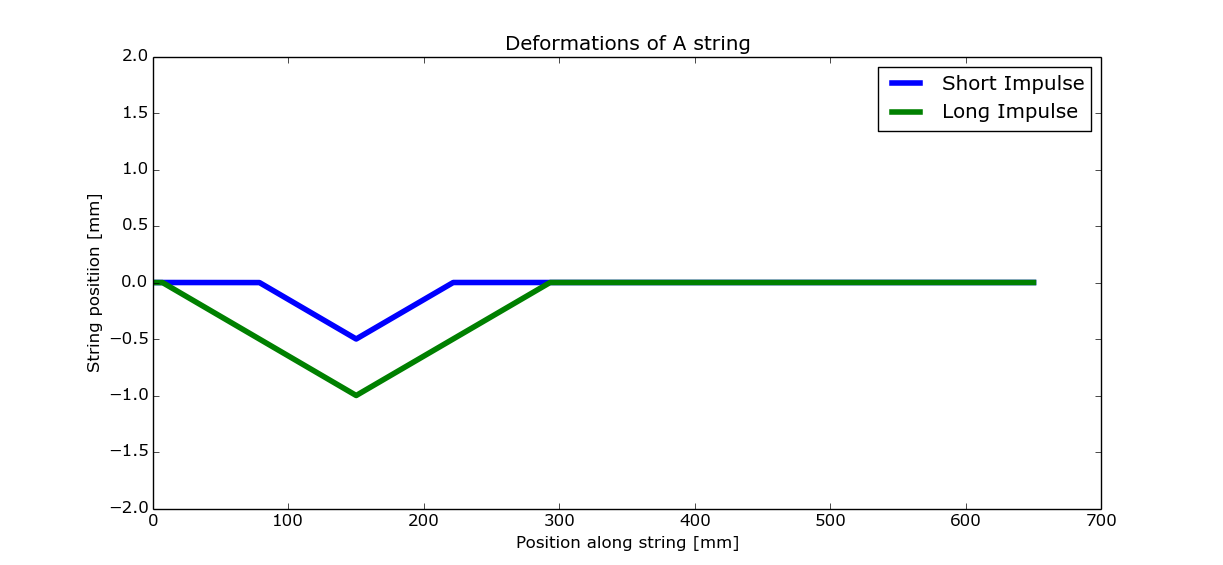
With these assumptions the maximum displacement of the string due to the pick is about 1mm -- this seems reasonable (at least of the correct order of magnitude). The linear shape of the tent used here is a bit of an idealization, in a real string there would be smoother curvature, but the fact that the portions of the string further away are unaffected is real -- due to the finite speed of propagation along the string. The details of the shape of the tent is less important than that it has finite extent.
The frequency content of this pair of initial conditions shows the expected result.
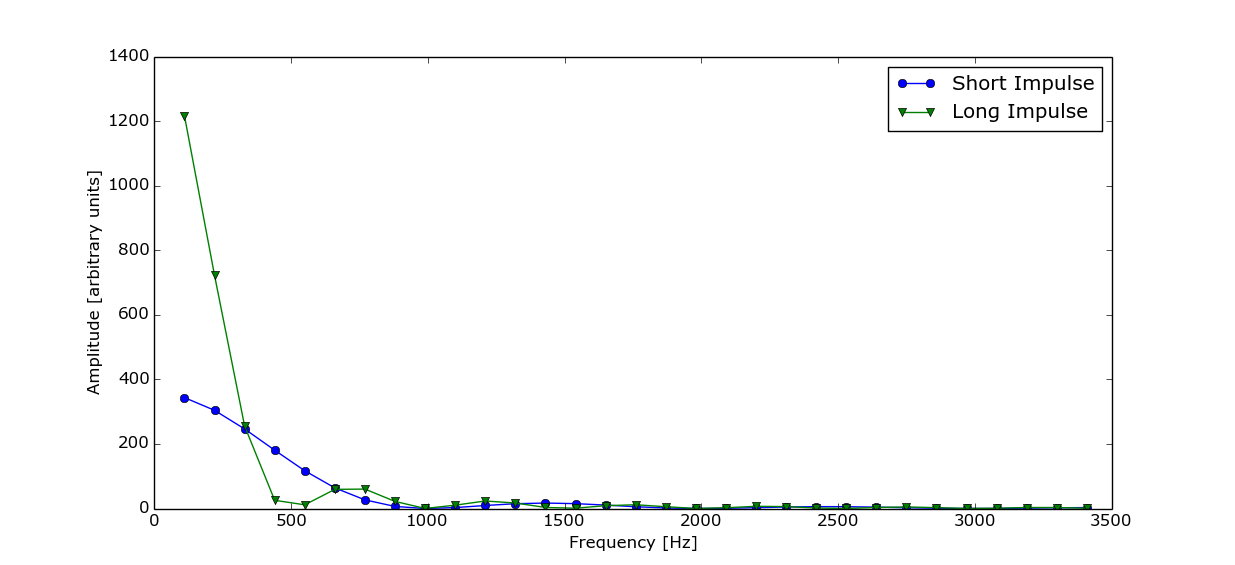
One defect of this analysis is that I've assumed that even though the string was deformed it was otherwise at rest. However, similar to the details of the deformation itself, the details of how fast the points on the string are moving is less important in this qualitative analysis than the fact that the region where the string is moving is bounded by the same (wave-speed-in-string)*(grip-time) distance.
The preceding analysis compares favorably to the results in Fundamentals of Musical Acoustics (A. Benade 1976) Chpt. 8 (which I didn't have handy at the time it was written). His summary is (paraphrased):
Vibrational modes whose period is more than 2x the impulse time are excited in almost exactly the same wayas by a hypothetical hammer that strikes and rebounds instantly.
Modes whose period is about 2x the impulse time are excited about 1/2 as much as by the ideal (instantaneous) hammer.
Modes whose period is less than the impulse time receive almost not excitation.
i.e. for 1/1000th of a second impulse, no frequency content above around 1000Hz.
Chapter 7 of the same also discusses the difference between "displacing" the string and "hitting" the string; the latter excites more higher modes. The portion above neglected this component. One can model the excitations induced by pulling the string some transverse distance, at some point along its length, holding it, and then letting it go. Although the details of how much each mode is excited depend on where along the string you've pulled, the general trend is that the amplitudes are proportional to 1/n^2 where n is the order of the partial. The claim is that when a string is struck by something hard (and instantly bounces off) the general trend of the amplitudes of the overtones is that they decay like 1/n -- i.e. striking the string causes more excitation in higher frequency modes than displacing and releasing the string.
We can see how this relates to pick thickness. A thick pick manages to push the string more, so there is less energy in the higher overtones. A thin pick strikes the string, then (nearly) immediately gives way -- behaving more like an instantaneous strike. Of course any real pick stroke is some intermediate between the idealized extremes of "pure displacement" and "pure strike" types of excitations.
I am not claiming that "grip time" is the sole determinant of the distribution of excitation across modes for any kind of pick, only that when considering the differences between picks of different thicknesses, but otherwise the same in terms of shape and material, the "grip time" is an important consideration.