Is there any scientific reason why three notes are seen as required to create an 'unambiguous' harmony?

Question

To me, three-note harmony works brilliantly with the diatonic scale and I can see why it became a common part of musical vocabulary.

Nevertheless, taking a step back from that particular tradition, it has never seemed obvious to me why three is seen as a 'magic number' here in a more general theoretical sense; and yet people frequently speak of e.g. two-note harmonies as 'ambiguous', or 'suggestive' of three-note harmonies, even when there seems no contextual reason to presuppose three-note harmony.

Is the tendency to see two-note harmonies as 'incomplete' simply result of people getting stuck in a certain set of cultural habits? Or is there a basis in physics / psychology why adding that third note suddenly creates a qualitatively different effect?

Answer 1

In short, no. There is no scientific reason why three notes are required to create an unambiguous harmony.

This is a self-fulfilling question in the sense that we already "define" harmony (implicitly) in terms of 3-, 4- or sometimes 5-note chords.

In a similar post we discussed here, chords (whether 2 or 3 or more notes) are contextual.

I'm sure there's some ingenious musician out there who could or will write a two part "harmony" that at times makes the "third note" implicit or at other times takes you places you were completely unsuspecting of, that couldn't have been done with three notes.

Another fundamental question to ask: were chords invented, or discovered? We can assume that melodies came to humans first, and then harmony and chords. So the question is, if we were to listen to melodies from other cultures say 3 or 5 thousand years ago, a) could we put chords to them and b) would the singers respond by saying "that is exactly what the song is meaning, you've just completed it"?

I'm also thinking to myself that probably most (but not all) calls esp. bird calls in nature are two notes..

Answer 2

It seems to me that, since three notes are required to spell out a chord, that would be the reason for the ambiguity. Lets take two notes E - G.

I would argue this is ambiguous because by itself it probably would make me hear an e minor harmony if it was spelled out low E high G (somewhere). Turn that around and maybe not.

However, C - E - G would be a C chord. E - G - B would sound like e minor. A - E - G would probably make me think of A7.

I would say that two notes can establish a harmonic progression just fine, just listen to any beginner Bach transcription for classical guitar and you can hear it. However you could easily disrupt that by adding in a third note (Easy Jazz Bach transcriptions for Classical guitar :) ). That third note would have the effect, I believe, in solidifying a harmonic progression that was otherwise ambiguous.

I think that above those three notes, it would be much more difficult to add in notes and actually create the impression of altering the harmonic progression, instead I think it would just end up being colored with extended harmonies.

Answer 3

Layers of Differences

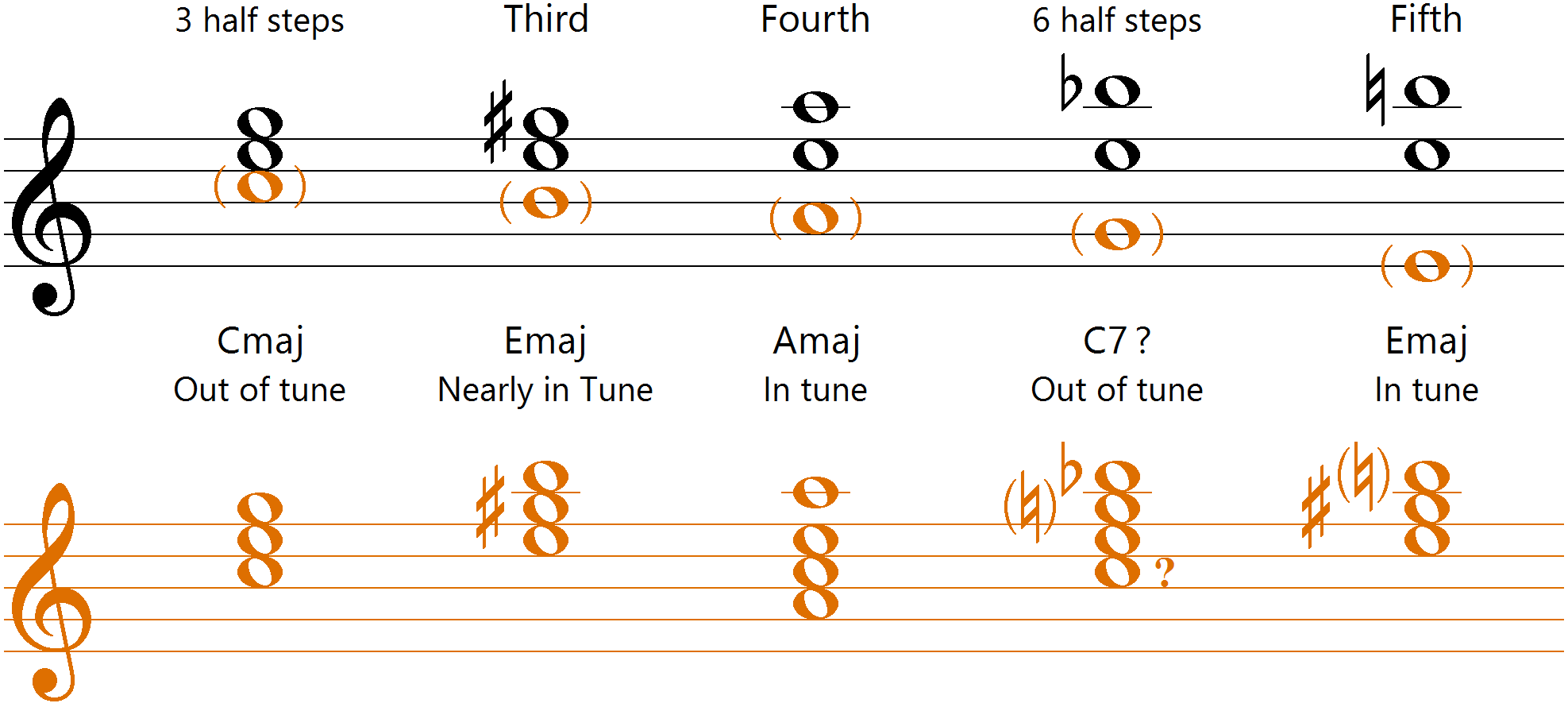
If someone were to ask, "what's that chord?" or "what's that harmony?" we could answer with varying degrees of specificity. The difference between CMaj and CMaj6 is much less than the difference between CMaj and Cmin. This suggests layers of distinction, and at the bottom lies some 'most fundamental' way of distinguishing chords. What is this most fundamental difference? The 3rd and 5th. But we need the root, too, so that we know which notes are the 3rd and 5th. This sets the requirement at 3 notes to create a definite chord: the 1st to establish the root, and the 3rd & 5th to define the chord quality.

The Role of Harmonic Function

Why are a CMaj and CMaj6 (or CMaj7 and CMaj9) so similar? I think it's because of harmonic function. They play the same role in harmony and are largely interchangeable. That's not to say that one won't be a better choice than the other--there will be scenarios where, e.g., CMaj6 does not complement the melody or resolution as well as CMaj. But even in those cases, wile the substitution may degrade the music, it can nonetheless be made without changing the underlying harmonic function of the chord. By contrast, changing a CMaj chord to a Cmin chord or to a Cdim chord would incur a (usually dramatic) change in the chord's function.

Why This Is So Limited

So the quality of a chord depends on the 3rd and 5th, because those notes largely determine and distinguish between different harmonic functions. But of course, this is an extremely limited statement, because harmonic function can vary from culture to culture, genre to genre, and time period to time period. Many people who say "a chord must have a minimum of 3 notes" actually mean something much narrower and would reject the idea that the notes C-C#-D form a definite chord. Really, when we espouse this criterion, we're working squarely in a particular Western tradition. We could easily imagine a musical tradition tens of thousands of years in the past where every single progression was I-vi, and the only difference between songs was the melody/chant. In that scenario, the 1st and 3rd would be sufficient to define a chord. If fewer types of chords exist, then less information is needed to distinguish them. We could even imagine a musical tradition that evolves so that, e.g., the V chord is always played with just two notes. Any time we hear that 2-note chord, we immediately know that it's a V chord. (I would imagine there are real-world examples to illustrate my point, but my knowledge is far too incomplete to posit what they may be without risking cultural insensitivity.)

Why Is It So Prevalent?

So the 3-notes = definite chord approach has obvious limitations. Yet, it is incredibly ubiquitous (at least in the West--maybe elsewhere), and for this reason, it has tremendous explanatory power. It is ingrained to a point where it's tempting to think of the 3-note requirement as a fundamental truth. Yet it can't be fundamental, because nothing in physics or psychoacoustics would preclude the creation of a simpler musical tradition where (a) 2-note chords were assigned specific and unique functions compared to 3-note chords, or where (b) few enough chords exist that they can be distinguished using only 2 notes, or where (c) chords never have more than 2 notes.

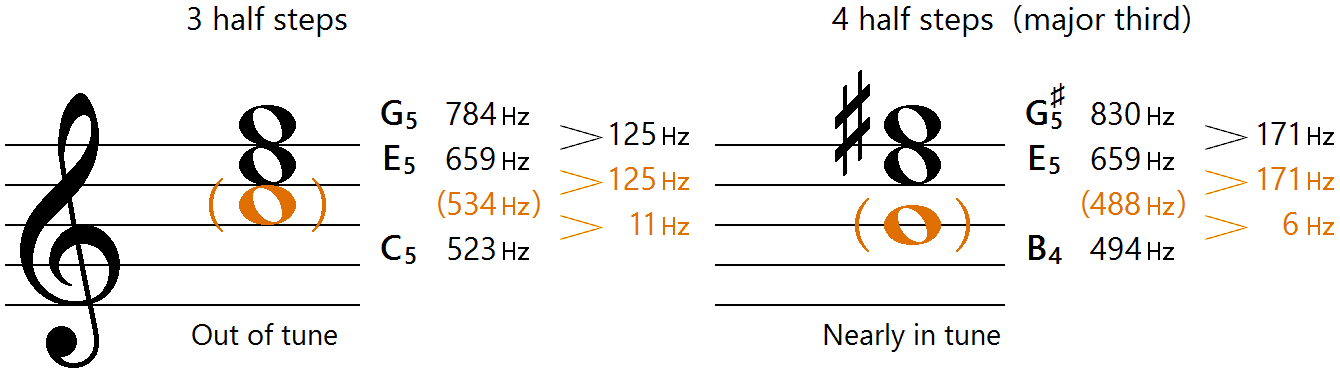
I don't know why the triad is so prevalent as a fundamental harmonic structure. I suspect it's a combination of biology + instrumentation + historical tradition. When the voice speaks or sings, we produce harmonics. The simplest single-note instruments similarly produce harmonics. As musical traditions are constructed throughout history, they begin with the human voice and simple instruments, and from there they build up in complexity. But the familiarity of the harmonic series might have suggested routes for the ever-increasing complexity of a musical tradition. At least, it's not a stretch to imagine that the first person ever to harmonize simply sang what they already were hearing: higher pitches from the harmonic series. There would be a positive feedback loop from this development in a musical tradition: chords drawing from the harmonic series would feel more immediately familiar, and in general humans tend to enjoy music/sounds that are more familiar. The same way that new-born babies recognize their mothers' voices, the harmonic series might have possessed an immediate biological advantage due to its familiarity in the voice and in the first single-note instruments. So it seems reasonable that chords would develop around the 1st, 3rd, and 5th, and that this might be one of the earliest things to become codified in the development of a musical tradition.

Note: in some ways, this feels like a "just-so story," and there are surely limitations to this approach. For example, the major 3rd doesn't appear until we reach the 5th harmonic, so perhaps this argument couldn't be extended to notes beyond the triad because harmonics above the 5th are simply too weak to hear.

Answer 4

Some two-note harmonies indeed have “a scientific basis for why adding a third note suddenly creates a qualitatively different effect.”   Three effects that occasionally come into play can be better understood after a mention of combination tones.
1.  Timbre   (a subtle factor)
2.  Intonation (a significant factor)
3.  Tangibility  (a less scientific factor)

Many two-note harmonies go beyond implying a third note to actually creating an audible illusion of three-note harmony. The result can create both problems and opportunities, as introduced nicely in the weblog A Mind for Madness.

Mathematical Music Theory 3:   Combination Tones
[ . . . ]
Composers such as Bach were intimately familiar with this phenomenon. Rather than have it do unexpected things to his compositions, he used it to his advantage. When he wrote two part inventions there would only be two melodies on top of each other, but due to combination tones it sounded much more fleshed out as if many more parts were being played. He would know that in parts where he wanted forward motion he would use unstable forms of intervals and where he wanted resolution he would use the stable forms.

When things go poorly, an actual played third note can rescue a well-intentioned two-note chord gone bad, mainly by overshadowing disruptive effects.

1.  Timbre can be disrupted, subtly.   Combination tones are bland, like featureless sine waves, which can sound intrusive when the original two-note harmonies strive for exquisite timbral blends.

Moreover, these tones will appear with different strengths and blends at different intervals during a two-note harmonic passage, like a third player who keeps switching instruments and styles.

2.  Intonation can ruin a chord.   An illusory third note (combination tone) is sometimes sorely out of tune with the chord it creates. Here is a demonstration that seems to work on every piano, whether acoustic or electronic. Play just the two top notes of each chord and listen for the third (parenthetical) note. The second staff summarizes the resultant chords.

These examples are in a range where combination tones are strong. Exact pitches at play in the first two intervals help understand why they are out of tune to different extents.

The 3-half-step harmony of E₅ at 659 Hz and G₅ at 784 Hz creates an illusory note at 534 Hz, which is separated by the same pitch difference. That new pitch is near enough to C₅ at 523 Hz to form a C major C-E-G triad but misses by enough to sound blatantly out of tune.

The 4-half-step harmony of E₅ at 659 Hz and G^♯₅ at 830 Hz works out better. One reason is that the illusory note at 488 Hz is relatively close to the 494 Hz B₄ that would be part of an inverted E major B-E-G^♯ chord. Another reason is that the 171 Hz pitch difference is relatively close to E₃ at 165 Hz, the fundamental note of that chord.

3.  Tangibility, for lack of a better term.   As audible as combination tones can be, they still feel different from played notes. Their incompleteness can unsettle music that is meant to be calm.

This is a minor point, admittedly, but has an irresistible visual analog. Do you sense faintly-dark blobs where they don’t exist in some corner gaps of this Hermann  illusion?

And, before long, aren’t you relieved by places where those blobs actually exist?

Further Reading
Wikipedia: Combination tone
Music SE: Classical examples of a ‘fifth voice’ or ‘ghost soprano’
Music SE: Is it possible to create the illusion of a sub-harmonic?
Encyclopædia Britannica: Combination tone
Encyclopædia Britannica: Noise —The ear as spectrum analyzer
A Mind for Madness: Mathematical Music Theory 3: Combination Tones