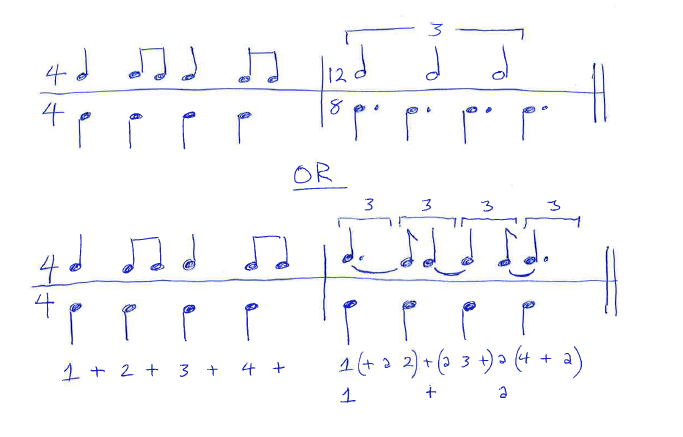
Polyrhythm 4:3 in 3/4 meter can be "inverted" to 3:4 in 4/4 meter, like this...
...the notation is easy enough to write, because we can use tuplets to gloss over the division problem.
Converting the 3/4 meter notation pattern to a counting grid I get...
3 |x| | |x| | |x| | |x| | |
4 |x| | | |x| | | |x| | | |
1 a 2 & 3 e
...the bottom line, under the x's, is how I count it out loud.
But if I convert the 4/4 meter notation to a grid, the uneven division problem is apparent, and I run into a counting problem...
4 |x| | | | |x| | | | |x| | | | | |
4 |x| | | |x| | | |x| | | |x| | | |
1 2 e 3 + 4
...the upper part isn't evenly spaced. Obviously the problem is 16 boxes on the grid can't be divided evenly by 3.
1st question:
If the 4 tuplets in 3/4 are timed to the exact same speed as the straight quarter notes in 4/4 will the other parts with 3 notes have the exact same timing regardless of which meter is used? I think the answer is yes, but I don't know how to prove that.
2nd question:
More important to me is how to count the pattern in 4/4 meter. It seems important to me to be able to count this pattern with either the 3 or 4 note base meters. If the triplet group is encountered in a 4/4 meter setting, I need to know how to count it while keeping a solid 4/4 count. Even if the 3/4 and 4/4 patterns are mathematically equivalent, I need some practical way to count it in 4/4.
I suppose the counting in my 4/4 example is close enough to be acceptable, but I don't want to practice it if there is a better way to count it.
Also, I understand that 4:3 polyrhythm in African music is normally written with a compound meter so so in that sense trying to count the pattern in 4/4 meter is contrary to that tradition.
EDIT
I'm adding this after reading the answers and feedback.
Is this the better way to do it? Either use 12/8 meter and make the tempo of the quarter note equal to the dotted quarter note, or use triplet eighths with ties.