How does any given major key contain only 1 tritone?

Question

Understanding the Fundamentals of Music (2006), by Robert Greenberg, B.A. in music (magna cum laude) from Princeton, Ph.D. in music composition from the University of California, Berkeley. p. 54 of the Lecture Transcript.

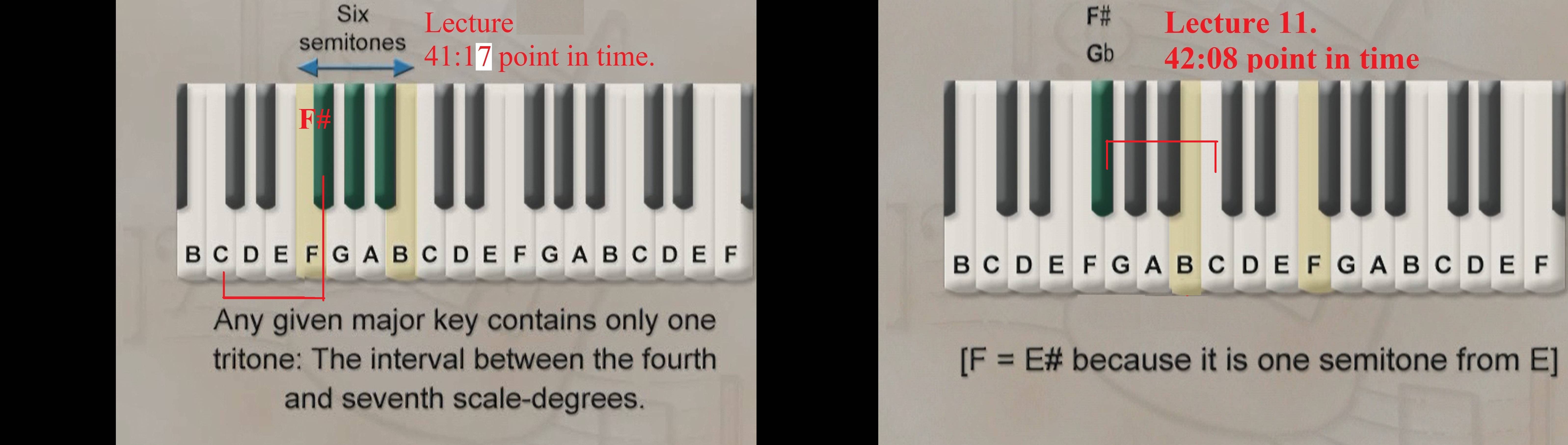
Any given major key contains only one tritone. It's the interval between the fourth and seventh scale-degrees. In the key of C major, for example, the tritone occurs between an F (the fourth scale-degree) and a B (the seventh scale-degree). Now, because there are 12 different major keys and only six different tritones, the tritones are doubled up: the same tritone will serve two different major keys. So, what major key does C major share a tritone with? The answer: the key of F# major, the major key farthest away from C major, the major key a tritone away from C major! My friends, keys a tritone apart will share the same tritone! (For our information, in F# major, the tritone occurs between B, which is the fourth degree of F# major, and E#, which is enharmonic with F, which is the seventh degree of F# major.) Dang, don't we just love the chromatic collection! The metaphors it presents us with are endless: The end is the beginning, the beginning is the end; opposites unite into singularities and singularities become opposites; all points are connected within the continuum. It's a fantastic system!

Why can a given major key contain only 1 tritone?

1. I don't understand why the "only one tritone" must be "the interval between the fourth and seventh scale-degrees"?

2. I added to Greenberg's figures. In the left figure, why can't C-F# be another tritone for C maj? In the right, why can't F#-C be another tritone for F# maj?

Answer 1

The lecture transcript isn't particularly clear unless you already have an understanding of the topic.

What is being presented is this:

in the major scale pattern, the tritone (three whole steps between notes) only occurs between the 4th note and the 7th note of the scale, exampled by in C major the jump between F and B (f-g-a-b, three whole steps).

     1 2 34 5 6 78
     C-D-EF-G-A-BC
           w w w 

He then goes on to explain that even though we can create a major scale pattern off of any of the twelve tones, because of the nature of the tritone it only occurs between six note parings (he really means tones, not notes) in the twelve possible keys.

Keys a tritone apart share the same tritone (in pitch, not note sequence, he doesn't make that clear).

For example if I am in the key of C major, the tritone above the tonic is F#, which isn't in the scale, it is the note between C Major scale's 4th and 5th degree, or a tritone higher.

     C-D-EFF#G-A-BC
     1 2 34TT5 6 78

If I move to F# Major, the then tritone in F# Major is the 4th to the 7th again, or B to E#, which is the actual pitch of F, so the B - F tritone.

Looking at the two keys, C major and F# major they share the same pitch jump of the tritone F-B, B-F, even though they aren't named the same.

     1  2  34  5  6  78 
     F#-G#-A#B-C#-D#-E#F#
              w  w  w

The main confusion is probably that when considering the tritone we are talking about the specific frequency jump of three whole steps, independent of the enharmonic naming of the notes.

Answer 2

"Any given major key contains only one tritone. It's the interval between the fourth and seventh scale-degrees."

Perfectly correct, if we take 'any major key' to mean just the notes of a major scale. If we have only C,D,E,F,G,A,B,C to choose from, the only tritone is between F and B.

Yes, of course we can play a tritone above C, which will be F#. Very likely it won't even imply a modulation to another key, we will still, in a very real sense, be 'in C major'. I'm delighted that you recognise this fact! A lot of questions in this forum are based on the misapprehension that only 'notes in the scale' are 'allowed. But, in this case, we ARE talking JUST about the notes of the major scale

Answer 3

Perhaps one way to "see" the explanation is to rearrange the diatonic scale in fifths and then noting the fifths are all perfect except for one...

CDEFGABCD...

Rearrange that in fifths...

CGDAEBFCG...

...you can see that only the B to F is a diminished fifth, a tritone.

Personally, I think the observation should be applied to the diatonic scale rather than the more specific major scale, because then you include the various modes. For example, the Dorian mode contains only one tritone. The same applies for all the modes.

For what it is worth, minor key music has two characterisic tritones: the leading tone to the sub-dominant, and the supertonic to the (lowered) mediant.

---

EDIT

I didn't see your specific question...

In the left figure, why can't C-F# be another tritone for C maj? In the right, why can't F#-C be another tritone for F# maj?

Diatonically speaking you won't be in the key of C major if you use an F#, and you won't be in F# major if you use a C natural.

Of course you can play those tones and still be in the respective keys, but they will be chromatic. For the purpose of the discussion "in the key of..." means the diatonic tones of the key signature and not chromatic tones.

The major/minor key system is a mix of diatonic and chromatic tones. Chromaticism can take the form of chromatic embellishment or shifting modes/tonics. "Being in a key" can be thought of has applying on different levels global or local. For example, a sonata in C major might contain passage that temporarily shifts into D minor. Globally the sonata is in C major, but the passage is locally in D minor.

You in one sense your are correct. You can have the tritone C F# in C major using chromatic harmony like V43/V V V42 I6.

On the other hand, if we want to discuss only the diatonic tones of the key signature, the tritone C F# is not in C major.

Answer 4

Interesting question that made me think. I'm a guitar player who had someone hand me a Circle of fifths chart years ago to help me understand some things about music theory. Now I just pulled out my Circle of fifths chart and looked at it again to see that for any key chosen, I can look directly across (180 degrees) the chart and find the tri-tone for that key, and I counted them and I'm seeing twelve of them. One for each key. I don't wish to put up an incorrect answer, but it is my understanding that the tritone is the center tone of the Octave and the only tone that stays the same interval when inverted. It is three whole tones (steps) from the tonic and three whole tones (steps) from the Octave. That's the definition I understand and I'm figuring twelve of them. Where's the fallacy in my understanding?

Answer 5

Compare all the notes in the key of C with those same notes shifted by half an octave:

    C # D # E F # G # A # B C # D # E F # G # A # B C
                C # D # E F # G # A # B C # D # E F # G # A # B C

Now notice that the only cases where two "white notes" line up are the "B/F" and the "F/B".

No matter what the key is, there will always be exactly one pair of natural notes where each one matches with the other.

Answer 6

I added to Greenberg's figures. In the left figure, why can't C-F# be another tritone for C maj? In the right, why can't F#-C be another tritone for F# maj

You must understand the fundamentals here: In any given key, each note (musical letter) appears only once in its major scale.

F# does not occur in the key of C Major (it's not diatonic - belonging naturally to that key.). F is the perfect 4th in C Major - there is no F#.

C natural does not occur in the key of F# Major. C# is the perfect 5th in F# Major - there is no C natural.

Your guide here is the key signature: The key signature shows you exactly which notes are part of that key - diatonic - just read it. Key signatures are not some sort of secret code - they simply tell you what notes are diatonic to the key in question.

The key signature of C Major has no sharps or flats - that means that all 7 notes diatonic to the key of C Major are natural. So, no F#.

The key signature of F# Major has 6 sharps - every note is sharped except for B - that means that all 7 notes diatonic to the key of F# Major are sharp except for B. So, no C natural.

However, in key of F# Major, the note E# does occur, as indicated by its key signature -it's the major 7th in the key F# Major. (In most places E# is called F but technically speaking, it is sometimes more correctly referred to as E#, such as when spelling the F# Major scale).

Counting from B natural - the Perfect 4 in F# Major - to E#, the Major 7th, we get an Augmented 4th - one form of tritone. This is exactly the same as going from F, the Perfect 4th in C Major, to B, the Major 7th - again an augmented 4th.

Going from the Perfect 4th to the Major 7th is the only interval that results in a tritone - in this case an Augmented 4th - in any one major key.

Any given major key contains only one tritone

is entirely correct - it's simple math.

Try it now, using the key signature as explained, as you will see this clearly.

Answer 7

The tritone embedded in a diatonic scale also carries through all of the modes, from Ionian through Locrian. In the key of C, the tritone (F-B) is located in the 4th and major 7th degrees of the scale in C's Ionian mode, the 3rd and 6th scale degrees in Dorian, 2nd and 5th in degrees in Phrygian, and 1st and 4th in Lydian. It then flips to B-F in G Mixolydian,A Aeolean, and B Locrian. So although each key has but one tritone, that tritone is available in all 7 modes of that key.