Frequency ratio, Wavelength ratio, and chords

Question

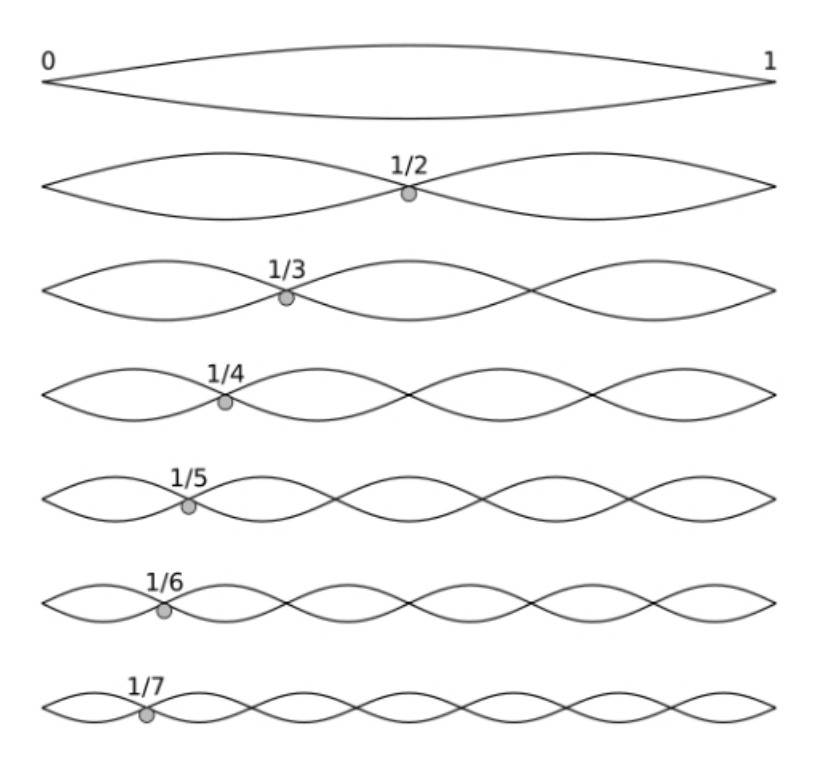
Here is a plot of list of wavelengths λ ($\lambda$, inverse proportional to frequencies f) of some strings, or sound waves.

Let us call the wavelengths as

λ1: λ2: λ3: λ4: λ5: λ6: λ7 and so on

= 1: 1/2: 1/3: 1/4: 1/5: 1/6: 1:7 and so on

Then their frequency ratios are

f1: f2: f3: f4: f5: f6: f7 and so on

= 1: 2: 3: 4: 5: 6: 7 and so on

Question

What are the famous (named) chords or note intervals known to produce harmonics?

For example, I know:

By searching online, I found a partial answer: https://pages.mtu.edu/~suits/chords.html

Hi wonderich - please re-read our tour and How to Ask pages, and limit yourself to 1 question in a Question post. phoog's answer is excellent, which is why I have rolled back to your original post, but please follow site guidance in future. — Doktor Mayhem, Commented May 29, 2023 at 9:52

phoog · Accepted Answer · 2023-05-28 22:17:56Z

Chords and intervals don't produce harmonics. Harmonics are present in musical tones in different proportions, an important part of musical timbre. When one pitch aligns with the harmonics of another, the two pitches sound more harmonious together. Beyond that, Stack Exchange discourages list questions.
Depending on what you mean by "noise," the answer is most likely either "all of them" or "none of them."
Different intervals are approximated by 12-tone equal temperament to varying degrees. The closest (other than the octave) is the perfect fifth. You can work it out yourself with a calculator that has a logarithm function. The usual way to do this is to calculate the interval's size in cents, where there are 1200 equal cents in an octave. The acoustically "just" perfect fifth, with a ratio of 3:2 or 1.5, is about 1.95 cents larger than an equal-tempered perfect fifth. The calculation is log(1.5)/log(2)*1200, which yields approximately 701.95. There are also lists, such as at Wikipedia and at Xenharmonic Wiki.