Chord pattern formula for constructing chords in a given major or minor key

Question

My question relates to the chord pattern formulas.

For this Example we are commonly given these two formula patterns as one of the steps to construct chords in a key. I have been self teaching/learning and can't seem to find any information on the web to explain how these patterns are derived?

For the Major scale = {Maj, Min, Min, Maj, Maj, Min, Dim} is used to build the chords in a key

For the Minor scale = {Min, Dim, Maj, Min, Min, Maj, Maj} is used to build the chords in a key

Anyone who can shed light on this part I would really appreciate. Thanks

Answer 1

Trying to encapsulate an answer that's concise and short!

Triads are basically 'stacked thirds'. That is, notes 1,3,5, and 2,4,6, and 3,5,7 etc.It is a fact that each root is from a diatonic note in that key. Thus 1,3,5 in key C is CEG; 2,4,6 is DFA, 3,5,7 is EGB etc.Some of those 'thirds' intervals are major (M), others minor (m). One has both intervals minor, (d) - diminished.

That gives a sequence of M m m M M m d, as others say.

Start the sequence on the last m - m d M m m M M - same order, different start point. So, really, one pattern!

Answer 2

For the purposes of this question, chords are most usefully defined as every other note from the root of the chord, with chords having their roots on each note of the corresponding scale.

Triads (3-note chords)

Given a major or minor scale, the chords, given by the scale degrees comprising each chord are

scale degrees	major scale example (C)	chord quality	minor scale example (A)	chord quality
1 3 5	C E G	major	A C E	minor
2 4 6	D F A	minor	B D F	diminished
3 5 7	E G B	minor	C E G	major
4 6 1	F A C	major	D F A	minor
5 7 2	G B D	major	E G1 B	minor1
6 1 3	A C E	minor	F A C	major
7 2 4	B D F	diminished	G2 B D	major2

Seventh chords (4-note chords)

scale degrees	major scale example (C)	chord quality	minor scale example (A)	chord quality
1 3 5 7	C E G B	major	A C E G	minor
2 4 6 1	D F A C	minor	B D F A	half-diminished
3 5 7 2	E G B D	minor	C E G B	major
4 6 1 3	F A C E	major	D F A C	minor
5 7 2 4	G B D F	dominant	E G3 B D	minor3
6 1 3 5	A C E G	minor	F A C E	major
7 2 4 6	B D F A	half-diminished	G4 B D F	major4

---

¹ _{It is conventional in a minor key to raise the 7th scale degree by a half step to create a leading tone. Thus the chord rooted on the 5th scale degree becomes major.}

² _{It is conventional in a minor key to raise the 7th scale degree by a half step to create a leading tone. Thus the chord rooted on the 7th scale degree becomes diminished.}

³ _{It is conventional in a minor key to raise the 7th scale degree by a half step to create a leading tone. Thus the chord rooted on the 5th scale degree becomes a dominant seventh chord.}

⁴ _{It is conventional in a minor key to raise the 7th scale degree by a half step to create a leading tone. Thus the chord rooted on the 7th scale degree becomes a fully diminished seventh chord.}

Answer 3

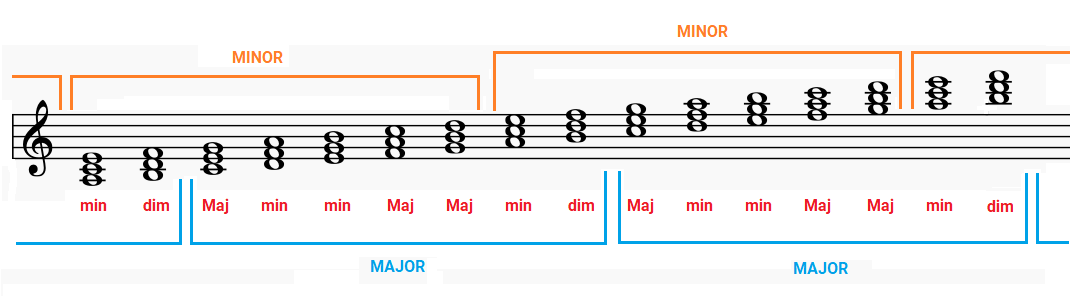
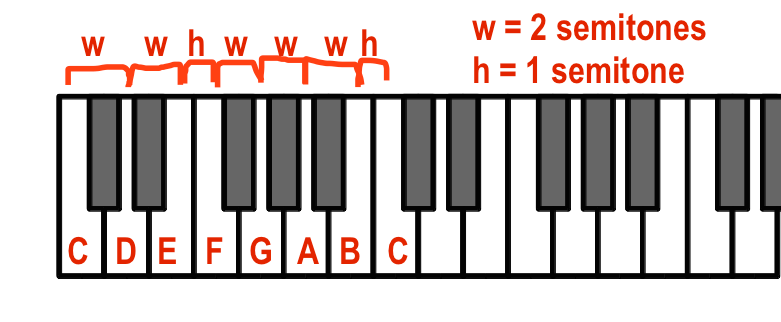
Let's look at diatonic notes starting from C. The white keys of the piano. These are the notes of the C major scale, and a similar geometry of intervals exists in all keys, it just starts from a different note and the white/black key distribution isn't so simple when you start elsewhere. The piano keyboard has been deliberately designed so that it is easy to play diatonic things in the keys of C major and A minor.

If there is a black key between two white keys, for example C and D, the interval i.e. pitch distance between those keys is one whole tone, denoted as "w" in the picture below. If there is no black key between two white keys, for example E and F, the interval i.e. pitch distance between those keys is one semitone. One whole tone equals two semitones, so you can call them Whole and Half. (Don't ask why the distance between E and F, and B and C is only "half" when it's a full scale step, that's just the way it is.)

Diatonic chords are constructed as stacks of thirds, i.e. by taking every other note of the scale. Scale degrees 1, 3, 5 are the first chord. Scale degrees 2, 4, 6 are the second chord etc. Such a jump, skipping over one scale note, is called a third. A jump encompassing three notes in the scale. C, D, E : three different note names, so a "third". The jump from C to G is ... C, D, E, F, G: five different note names, so it's a "fifth".

Here is the first such chord in the C major scale:

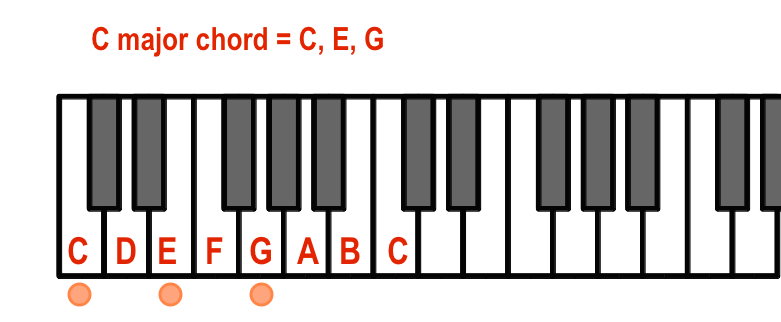
As you can see, it starts from C, and the first interval in the chord, from C to E, is two whole tones. Which is four semitones. 2+2=4. The note E is called the "third" of the chord. Yes, it's the second note of the chord, but it's the chord's "third". Sorry about that. The jump C-D-E is a third, but C-D-E-F-G is a fifth.

When a chord's third is four semitones, it is a major chord. When a chord's third is three semitones, it is a minor chord. Major = larger. Minor = smaller. Four is larger than three.

Let's take the second chord of the scale:

As you can hopefully figure out, the jump from D to F is three semitones. It's not actually rocket science, even though it seems to be so incredibly difficult to understand, based on the utter confusion people have over it. From D to E, there is a black key, so it is a whole tone, i.e. two semitones. From E to F, there is no black key, so it is one semitone. 2 + 1 = 3. Three semitones means a minor third, which means that the stack of two consecutive thirds starting from D is a minor chord.

Count the semitone jumps from D to F: ... 1, 2, 3!

In the D minor chord, the jump from D to A, just like the jump from C to G, is a fifth. And it's seven semitones, a "perfect fifth". So, what makes a major and minor chord different from each other is the third.

Now there's just the one different case, B diminished.

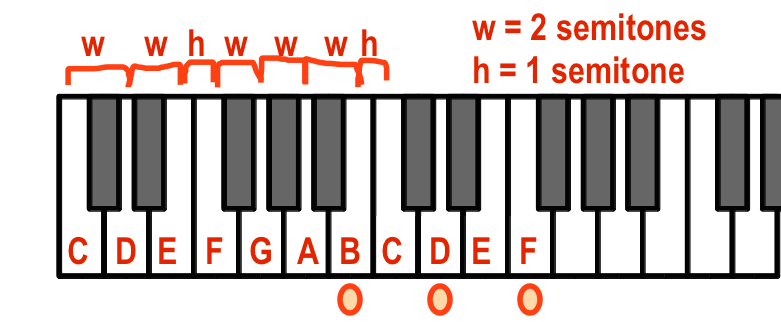
From B to D, the distance is three semitones. And from D to F, like we already saw, it is three semitones i.e. a minor third as well. A chord with two stacked minor thirds is called a diminished chord. The fifth in a diminished chord is only 6 semitones, as opposed to the 7 semitones of the major and major chords, and that's why it's called "diminished".

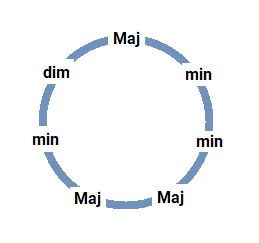
Ok. But where does the other list come from? If you start building the list of chords from the A note instead of the C note, you get the second list. The first one is a minor chord, A - C - E. Then a dim chord, B - D - F. Then a major chord, C - E - G. Etc.

Answer 4

The minor scale is just the major scale displaced by a 6th. It becomes clearer if we label the elements of the major scale below and then list the associated components of the minor scale.

Major scale = 1-Maj, 2-Min, 3-Min, 4-Maj, 5-Maj, 6-Min, 7-Dim

Minor scale = 6-Min, 7-Dim, 1-Maj, 2-Min, 3-Min, 4-Maj, 5-Maj

Answer 5

With the help of all the above contributors and especially @piiperi I would like to answer my own question as a way to demonstrate newly learned knowledge that you have all taught me and hopefully make it easier for others trying to learn this as well.

As peri stated the process can be confusing to a newcomer counting intervals and then relating them to thirds. The aha moment was when I studied peri's statement that major or minor is defined by deciding whether the 1st third in the triad is 4 semitones (A major) or 3 semitones (Minor) or 3ST on both sides of the middle third (Diminished).

I was still very perplexed and kept going in circles with my reasoning as I tried to understand how one would come up with the cord pattern from scratch.

                        {Maj, Min, Min, Maj, Maj, Min, Dim} 

I wanted to be able to take any note (Key) and then use it to build a family of Chords.

First I had to understand the pattern _W_W_H_W_W_W_H (Whole and half steps) and then realize this is the main chord building template. I knew the pattern as fixed on the keyboard, however I failed to understand the pattern must start in the key you are working with i.e. your whole step must start from the first to second degree in the scale you are working.

For Example:

To build chords in D Major, start with D in the first blank of the template then complete the scale. It is quickly apparent by looking at the whole and half steps there are errors needing to be fixed, therefore you proceed to make the required changes to match the pattern by raising a semitone where needed.

D W E W F H G W A W B W C H

Step 2: Correct them to match the whole and half step intervals

D W E W F# H G W A W B W C# H

Again Without Intervals Shown:

D,E,F#,G,A,B,C#

Step 3: Build Chords for all notes in the scale

DF#A, EGB, F#AC#, GBD, AC#E, BDF#, C#EG,

Step 4: Apply Major or Minor designations by looking at the intervals in the triad

Major Minor Minor Major Major Minor Diminished

For Minor scale you convert the major scale by lowering the 3rd 6th and 7th degrees by one 1/2 step. For a new student perspective it should also be noted that a degree should not be confused with intervals. A degree is simply a position in the sequence 1,2,3,4,5,6,7 for the scale. So in our D major scale 3,6,7 would be F#,B and C# therefore the D minor scale would be

D,E,F,G,A,Bflat,C

Chords can then be built and we can discover the Pattern:

                      {Min, Dim, Maj, Min, Min, Maj, Maj}

By evaluating the intervals in the triad as we did for the major scale above.

Answer 6

The patterns derive from the three note triads built on each tone of the gamut of letters A B C D E F G. You call these the diatonic triads...

...notice that the pattern is really one pattern of seven chords where major and minor are just segments within that seven chord pattern.

The seven chord pattern can theoretically ascend or descend infinitely which is represented visually more easily as a circle...

Answer 7

I tend to organize this using modes.

A diatonic scale has good distribution across the octave and (in some sense, both in terms of spacing and with respect to the overtone series highlighting thirds and fifths) naturally supports the notion of choosing every other note (thirds) to build a diatonic chord. These notes are then placed in parallel to finish the construction. I squint my eyes and see this as building harmonic elements by parallelizing melodic ones.

With that idea, we try taking a scale (melodic element) and putting it in parallel with other scales. We'll choose modes (relative to the root degree) for the scales and use the reasoning above to choose every other mode. Hence, we add modes in parallel according to some cyclic order of (1,3,5,7,2,4,6) to build progressions of diatonic triads, sevenths, etc... A triad example in C-major:

 I    ii   iii  IV   V    vi   viid I
-G----A----B----C----D----E----F----G- Mode=Mixolydian (5)
-E----F----G----A----B----C----D----E- Mode=Phrygian (3)
-C----D----E----F----G----A----B----C- Mode=Ionian (1)

From the intervals, we get an ordering of MmmMMmd chords being produced that repeat (i.e. it's cyclic). So, we can see for example that the natural minor case is already written in the example, we just start at the A on the bottom row (which represents aeolian and a parallel order of 6-1-3), making mdMmmMM.

Answer 8

A lot of these answers are only partially correct and some have incorrect information in them. I'll try to clarify.

It's important to understand that chord quality and construction is independent of scales. Yes, both are defined by intervals, but their construction is mutually exclusive.

A scale is a sequence of intervals. In western music, there are Major scales and three types of Minor scales. Each type has a unique sequence of intervals that define it (as many of the posts here discuss). A scale can become a Key when the root note is assigned. For example, the Major scale becomes the key of "C Major" when you define "C" as the tonic of it. But all of this is irrelevant to chord construction.

Chords are also constructed of sets of intervals. Chords are simply 3 or more intervals intended to be played in unison (or rapid sequence as in strumming a guitar). A Major chord is defined as the Unison, a Major 3rd, and the Perfect 5th (technically a Minor 3rd above the first Major 3rd). Technically, these are not intervals of a scale, though a lot of people think of them that way. They are simply the number of semi-tones (or half-steps, if you like) between the notes.

Triads are very specific types of chords using exactly the Unison, a Major or Minor 3rd above it, and a Major or Minor 3rd above that. A Major chord is a type of Triad, but a Seventh chord is not because it has 4 intervals. Some chords have upwards of 7 or more intervals.

Finally, it doesn't matter which scale you are using, the chord constitution will always be the same. When playing a C Major chord on a piano, you will always play the same 3 physical keys (the C key, E key, and G key) regardless of which scale / Key you are playing in. The only thing that would change based on the key (i.e. scale) would be the note names of those 3 keys, but the physical pitches will always be the same.

If you are looking for which intervals chords are constructed from, this page lists a couple of dozen chord types and their intervals: https://www.internetchorddatabase.com/Reference.aspx

Answer 9

I think it's important to understand the structure of the major scale and the answer given by Son of Fire makes this explicit. You are not really given a formula for the chords in a key in my opinion, you are given the definition of a key, and a formula for building a chord. From these two kernels the rest follow. And since major and natural minor are related it's no surprise that the set of chords is the same, just in a different sequence because natural minor scales and major scales are related, relative minor starts on the 6th degree of the corresponding major.

This follows for all the modes, as pointed out in other answers. If you ask what are the chords that naturally occur in Phrygian just start the major scale sequence on the 3rd degree and keep going.

It might be more interesting to look at melodic minor, or some other exotic, non-Western scale. The (jazz) melodic minor is W-H-W-W-W-H, e.g. A mm would be {A, B, C, D, E, F#, G#, A}. The triads that naturally occur here are: A-, B-, C+, D, E, F#dim, G#dim, A-.

Try applying the formula to other examples and you will become proficient at it and understand the relationship.