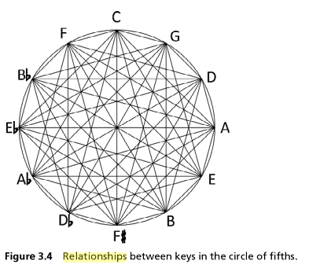
The author seems to be actually attempting to connect all the keys and it may be unintended that there are keys that are disconnected as in the paragraph after he starts talking about modulation and changing keys.
When and if the key of the music changes-- a process called modulation-- this will always be from on the circle to another... This can be more readily appreciated if we draw in the various relationships between keys within the closed circle. The result is a complex geometric diagram that precisely reveals a precise map of key relationships.
The picture is confusing as is and like you said not very well explaining the concept of why keys are related so let's take a step back in this to understand how keys are related.
In music theory we determine how closely related one key is to another by what notes they have in common. The more notes they have in common the more related they are. To keep things simple at first, we'll only consider major keys for now.
The basic pattern for a major scale in whole steps and half stets is:
WWHWWWH
When we use the pattern on with C as the tonic we get the notes:
C-D-E-F-G-A-B-C
When we use the pattern on with G as the tonic we get the notes:
G-A-B-C-D-E-F♯-G
When we use the pattern on with F as the tonic we get the notes:
F-G-A-B♭-C-D-E
As you can see, between both C and G there is only one note difference as C major has an F while G major has an F♯ so these keys are very closely related and between both C and F there is only one note difference as C major has an B while F major has an B♭ so these keys are also very closely related.
It should also not be a surprise that these notes are each a 5th away from C, the G being a perfect 5th up and the F being a perfect 5th down, showing how the pattern of the circle of fifths
Now, let's take a closer look at the difference between F and G which also aren't connected on the linked graphic. These keys have 5 of the 7 notes in common so they are pretty close, but it's not as closely related as they are to C.
Another thing to note is that the notes they have in common are G, A, C, D, and E. As you can see F, which is the tonic of F major, does not appear in G major, but G appears in both. So even they are pretty closely related, it's a little harder to go from G to F due to the F not being common in both then it is to go from F to G since the G is common in both.
This pattern continues as you go further in distance around the circle. So in the same manner, it's a little harder to go from C to Bb then it is to go from Bb to C and it's a little harder to go from D to C then it is to go from C to D, but they are still very closely related keys even though for some reason they are not connected in the picture you we show.
The keys that have the weakest relationship are a tritone (Augmented 4th/diminished 5th) apart. For C these keys would be Gb/F# which in the circle are the furthest away which is for a very. It should be noted however that even though they are the furthest away it's still possible to modulate to them which I kind of think the author wanted to point out that it's possible to go to any key from any other key with a bit of work and carefully crafting a progression to get you there.
Conclusion:
So as far as the key relationships, the closer in distance in the circle they are the more they are related. You can get to any key from any other key, but the closer the keys are the less effort and natural it is to modulate to which may have been what the graphic was trying to depict, but came across pretty poorly. I drew up a picture to represent how closely related keys are based on distance. The numbers on the line represent how many notes the keys have in common.
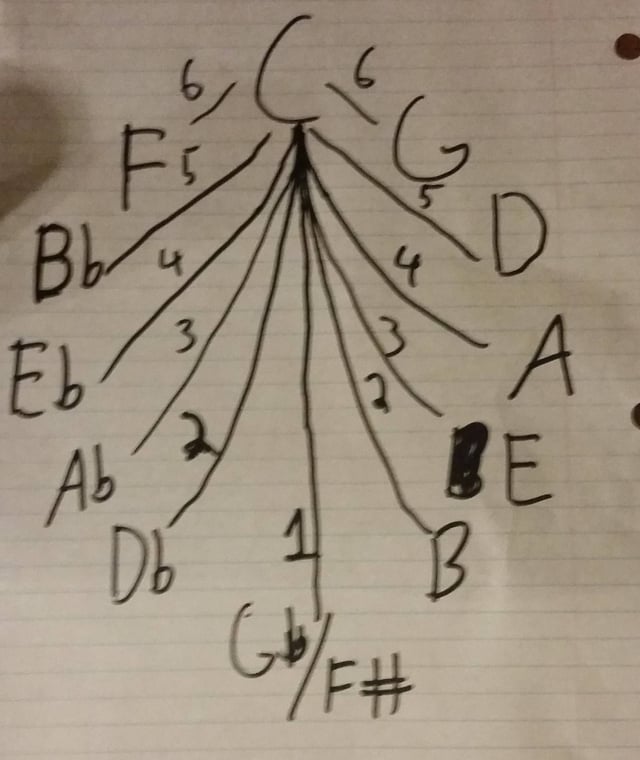
Not the prettiest graphic, but it should visualize how related the keys are in a similar manner as the author tries to get across.