Curious as to why this substitution of the root for a ♭VI chord works. It's pretty standard but I don't get the theory of it. I recently saw it being used in the context of a ii - V - I to delay the final resolve of a song. Specifically the following:
|| A7#11(13) Amin9 | C/D D7b9 | EbMaj7♯11(13) ||
I've seen other progressions walk up to resolve as such: ii - V - ♭VI - ♭VII - I
What is the theory surrounding a ♭VI substitution like this?
I recently saw it being used in the context of a ii - V - I to delay the final resolve of a song. Specifically the following:
|| A7#11(13) Amin9 | C/D D7b9 | EbMaj7♯11(13) ||
I suppose you're asking about the D7b9 resolving to Ebmaj7 rather than to a tonic I chord rooted on G.
This should be a straight forward question and answer, but it's complicated by all the complex details of the example progression - lots of chord extensions and mode changing - and the notion that V ♭VI is a "root substitution."
Progressions V ♭VI or V vi are simply deceptive progressions. The idea is commonly covered in harmony textbooks. Basically, the "deception" is instead of V going to the tonic I or i, it goes to the chord of the submediant, vi or ♭VI. The "working" of that progression is the tritone of scale degrees ^4 ^7 resolves to ^3 ^1 in both progressions (V I and V vi of the minor mode versions) but the root of the second chord changes.
Using the basic idea of the example progression, it would be progressions the following where the tritone resolution is in the treble clef...
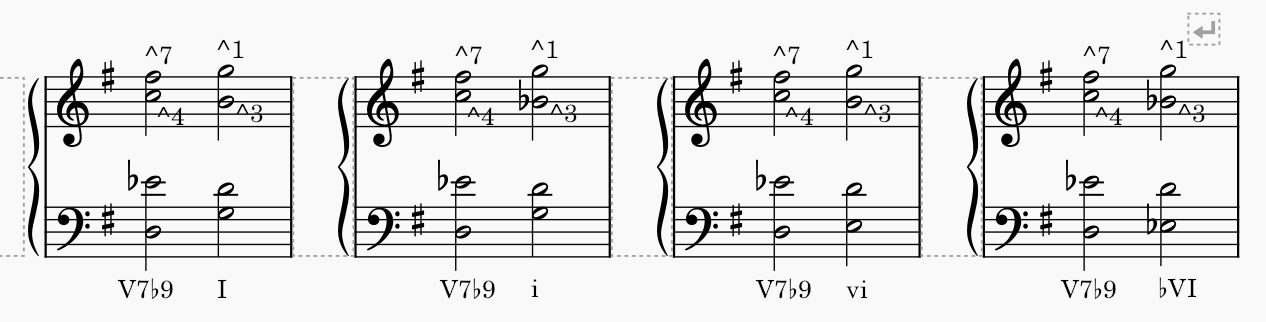
...those are not meant to be good jazz voicings. The idea is only to show the theoretical "workings" of the progressions which is the resolution of the tritone inward to a third or outward to a sixth.
It seems worth pointing out those progressions don't involve a root substitution but simply a root change, because it made the OP's question hard to understand.
The root of I (or i) is scale degree ^1. When the progression moves to ♭VI instead of I the root becomes scale degree ^6, but importantly the ^1 is still present in the ♭VI chord. Notice how G is still present in the deceptive resolution, but it becomes the chord's third...
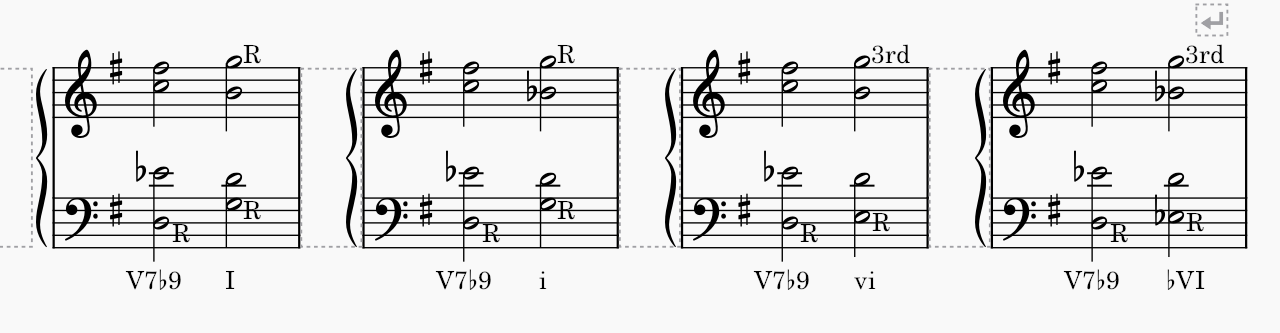
If anything is being substituted in the chord of resolution it is scale degree ^5 (the chord's fifth not root) being substituted with scale degree ^6. That nuance is probably more apparent when the chord of resolution is a plain triad, which is the basis of the "working", rather than jazz seventh chords.
It can operate in three ways:
bVI from the parallel minor rather than the native vi.bVI - bVII - I example, where the bVI leads to the dominant-function bVII.b6 can drop to 5 (as in a an actual plagal cadence), and the b3 can rise to 3.I think you're asking about the chord A♭ (or A♭7) followed by chord C, for example, in key C.
If you are, there's only the C note in common, all the others are non-diatonic, which makes the listener pay more attention, expecting diatonics.
So, C, with CEG, and A♭7 with A♭ C E♭ G♭. It can come across as a key change - that chord is the V of C♯, a quite common key change up a semitone, which obviously doesn't happen.
Notes like to be heard to resolve cleanly, and one semitone is often the cleanest path to take. The E♭ will move to E, while both the G♭ and A♭ converge to the G.
It's almost a plagal cadence, which would be F>C, or Fm>C, as Fm is the relative of A♭, so using the parallel key, as Aaron states.
To me the theory behind why the V7-bVI works is that the tritone of the V7 chord, resolves in exactly the same way as it does in a V7-i. The bVI comes from the parallel minor. Using your example’s key of G, the F# leading tone of the D7 resolves up to a G and the C resolves down to a Bb. The notes G and Bb are in both the bVI (Eb) and the i (Gm) chords.
